Introduction
Electrons encounter resistance when they go through a conductor because of the molecules’ attraction forces. The nature of the material determines how much of this resistance is provided. The resistance of the material determines how much electricity flows as a result of voltage differences. Ohm’s law relates electric current (I), voltage (V), and resistance such that,
V = IR
Electrical resistors are devices that provide resistance to an electric circuit. The zigzag symbol in an electrical circuit diagram stands in for a resistor.
System of Resistors
Systems of resistors can be arranged in series or parallel.
1. Resistors in Series Arrangement:
The resistors are arranged in this configuration along the current’s path, one after the other (end to end). As the current passes through the first resistor, its output current enters the second resistor as an input, and the second resistor’s output is then transferred to the third. The equivalent resistor, whose total equivalent resistance is simply the sum of the individual resistance of all the resistors linked in series, may replace all the resistors in a circuit. The equivalent resistor’s formula is:
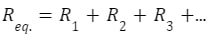
Each resistor in a series circuit receives the same amount of current, and the voltage across each resistor varies proportionally to its resistance.
The total voltage of the circuit is equal to the sum of the voltage across each resistor when the total current, I, in the circuit is multiplied by both sides of the equation.
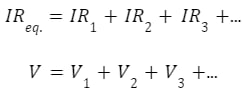

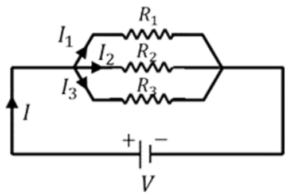
2. Resistors in Parallel Arrangement:
All the parallel resistors in this configuration share an input lead and an output lead, i.e., they are connected across each other. Each resistor in a parallel combination has the same voltage across it, which is the same as the circuit’s overall voltage. At a junction, the electric current is split based on the resistance of each resistor. At the output junction, the whole output current is combined once more and flows through the circuit.
Equivalent resistance in parallel is given as follows:
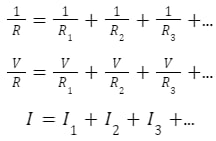
Since the total voltage on each side of the equation is the same, the voltage across each resistor is also the same. We can see that the circuit’s total current, I, equals the sum of the currents flowing through all the resistors.
 Summary
Summary
Small electrical components known as resistors provide resistance to the passage of electricity in an electric circuit. A circuit can link many resistors in series or parallel configurations. If many resistors are replaced with a single resistor that has the same resistance as the combination, the equivalent resistance of that resistance is the same as the resistance of the series and parallel combination of resistors. The combination formula for series resistors is, Req.=R1 + R2 + R3 +…, and for the parallel combination 1R = 1R1 + 1R2 + 1R3 +…
Frequently Asked Questions
1. What are the Factors on which the Resistance of an Object Depends?
Ans: An object’s electrical resistance is determined by the characteristics of its material and form. The formula takes into consideration these elements:
R= ρ (l/A)
Where A is the cross-sectional area of the material, Rho is its resistivity, and l is the length of the material through which electricity is flowing.
2. What is Electrical Conductivity?
Ans: The inherent capacity of a substance to carry electricity is known as electrical conductivity. It shows how readily electricity can go through the substance. The symbol for conductivity is (sigma), which is just the reciprocal of resistance such that: σ = 1/ ρ
Conductivity equals. Like resistivity, which is a broad attribute of a material that depends on its size. Air is a superb insulator with very low conductivity, whereas metals are typically good conductors with high conductivity and low resistance. Even at temperatures close to absolute zero, superconductors exhibit conductivity.
3. What is the SI unit of Resistivity and Conductivity?
Ans: The most used system of measuring in contemporary times is the SI unit or the International System of Units. The globe uses this contemporary metric system, which is utilised in all languages.
The SI unit of resistivity- ohm metre (Ω.m).
The base SI unit of resistivity- kg.m³.s−³.A-².
The SI unit of conductivity- siemens per metre (S/m).
The base SI unit of conductivity- kg-¹.m-³.s³.A².