Tag: Perpendicular

Introduction

We come across several items of various shapes and sizes in our daily lives. Many items have three sides, whereas others have four, five, and so on. Some forms have equal sides on all sides, whereas others do not. Consider our laptop screen, deck of cards, tabletop, chessboard, carrom board, and kite as examples. What feature does each of these shares? Each of them has four sides.

A quadrilateral is a flat shape with four straight sides. The terms Quadra and Latus combine to make the word quadrilateral. Quadrilaterals are simply shaped with four sides since Quadra means “four” and latus means “sides” in Latin.

One definition of a quadrilateral is a closed four-sided shape. There are many different types of quadrilaterals, including square, rectangle, rhombus, trapezium, kite, etc., supported by the characteristics of sides and angles.

Quadrilaterals

A quadrilateral is a polygon with at least and at most four sides, four angles, and four vertices.

Just like every other polygon, except triangles, the quadrilaterals are also divided into two subcategories,

1. Concave Quadrilaterals: These quadrilaterals have one diagonal passing through outside of the body of the quadrilateral. The following image shows one example of such quadrilateral 
2. Convex Quadrilaterals: These are the normal quadrilaterals, that have all the angles less than \(180^\circ \) , and both the diagonals are always contained within the quadrilaterals.

These are divided into \(2\) more categories

1. Regular: In these quadrilaterals all four sides and all four angles are equal to one another. The only regular quadrilateral is Square.
2. Irregular: In these quadrilaterals, all four sides or all four angles are not equal to one another. There are many irregular quadrilaterals, such as, Rhombus, Rectangle, Trapezium etc.

Properties in Quadrilaterals

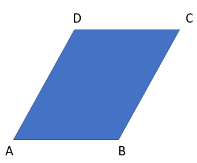
Above, we have a quadrilateral \(ABCD\) 

• This quadrilateral has \(4\) sides, and they are \(AB,BC,CD\) and  \(DA\) .
• This quadrilateral has \(4\) vertices, and they are \(A,B,C\) and \(D\) .
• This quadrilateral has \(4\) angles one at each vertex.

Angle Sum Property of a Quadrilaterals

The Angle Sum Property of a Quadrilateral states that the sum of a quadrilateral’s four internal angles is \(360^\circ \) .

I.e., in above example of Quadrilateral ABCD, we have,

\[A + B + C + D = 360^\circ \]

Types of Quadrilaterals

In this section, we will discuss the different types of quadrilaterals and some of their properties.

Square

This is the regular quadrilateral, i.e., all four sides and all four angles are equal to one another. The diagonals are also equal, and bisect each other at right angles. By the property of regular quadrilateral and angle sum property, the angles of the square are right angles.

Rectangles

Rectangles have opposite sides equal and parallel, and all four angles in rectangles are right angles. The diagonals are equal, and they bisect each other but not at right angles.

Also Read: Exterior Angles of a Polygon

Rhombus

Rhombus’ have opposite angles equal, and all four sides in rhombus’s are equal. The diagonals bisect each other at right angles.

Parallelograms

Parallelograms have opposite sides equal and parallel, the opposite angles are also equal in a parallelogram. Diagonals bisect each other.

Trapezium

Trapeziums have only one property, i.e., they have one pair of opposite parallel sides. Other than that they can have any side lengths, any angles, and any diagonals.

Summary

This article taught us about quadrilaterals, including their kinds and qualities. A \(2D{\rm{ – }}shape\) with four sides, four vertices, and four angles is referred to as a quadrilateral. Quadrilaterals come in a variety of shapes, including rectangles, rhombus, squares, trapezoids, parallelograms, and kites. These all feature unique angles and side characteristics.

Frequently Asked Questions

1. Are all parallelograms rectangles? What about the other way around?

Ans. No, all parallelograms are not rectangles. Since to be a rectangle a parallelogram should have diagonals equal, they should also have all the angles to be right angles. Which is not the case for parallelograms. Whereas if we see it other way around, we have the pair of opposite sides parallel and equal to each other and the diagonals also bisect each other, thus all rectangles are parallelograms.

2. What are the properties of a kite?

Ans. Kite is a convex quadrilateral with pair of adjacent sides equal to each other and the diagonals are perpendicular to each other. Also, the diagonal between the equal sides bisects the other.

3. A square has the properties of all three, parallelogram, rectangle and rhombus. Justify this statement.

Ans. A square has following properties.

• Opposite sides parallel and equal. (Parallelogram)
• Opposite angles equal. (Parallelogram)
• Diagonals bisect each other. (Parallelogram)
• Diagonals are equal. (Rectangle)
• All the angles are equal to right angles. (Rectangles)
• All four sides are equal. (Rhombus)
• Diagonals are perpendicular bisectors of each other. (Rhombus)

Thus it is clear that the square has the properties of all, parallelogram, rectangles and rhombus.

Introduction

A straight line that intersects another straight line at a 90-degree angle is said to be perpendicular to the first line. The small square in the middle of two perpendicular lines in the figure represents 90 degrees, also known as a right angle. Here, two lines cross at a right angle, indicating that they are perpendicular to one another.

In contrast to sloping or horizontal lines or surfaces, perpendicular lines or surfaces point directly upward. An object is at a 90-degree angle to another if it is perpendicular to it. A pair of lines, vectors, planes, or other objects are said to be perpendicular if they intersect at a right angle. Two vectors are perpendicular if their dot product equals zero.

Perpendiculars

When two lines intersect at a right angle, they are said to be perpendicular to one another. A first line is perpendicular to a second line, more specifically, if the two lines intersect and the straight angle on one side of the first line is split into two congruent angles by the second line at the intersection. Since perpendicularity is symmetric, if one line is perpendicular to another, the other line is also perpendicular to the first. As a result, we don’t need to specify an order when referring to two lines as perpendicular (to one another).

Perpendicular Lines

Segments and rays are easily extended by perpendicularity. If a line segment AB and a line segment CD result in an infinite line when both directions are extended, then the two resulting lines are perpendicular in the sense mentioned above. Line segment AB is perpendicular to line segment CD and can be represented by the symbol AB ⊥ CD. If a line crosses every other line in a plane, it is said to be perpendicular to the plane. The definition of line perpendicularity is necessary for understanding this definition.

Perpendicular Theorem

According to the perpendicular line theorem, two straight lines are perpendicular to one another if they intersect at a point and create a pair of equal linear angles.

Assume two lines AB and CD intersect each other at O, such that ∠AOC = ∠COB, also since AB is a line, ∠AOC and ∠COB also form a linear pair.

Then, ∠AOC + ∠COB = 180°

Using ∠COB = ∠AOC

⇒ ∠AOC + ∠AOC = 180°

⇒ 2 ∠AOC = 180°

⇒ ∠AOC = 90°

Thus, since the angle of intersection is 90°, we can say that AB is perpendicular to CD and vice versa.

Also Read: Related Angles

Perpendicularity: Slope Formula

Perpendicularity is known as the mathematical condition that two lines need to satisfy to be called perpendicular. Mathematically, if two lines are perpendicular to each other, then the product of their slopes is negative unity.

For example, let two lines of slope . Then these lines are said to be perpendiculars to one another if their slopes have a product -1, i.e.,  

Equation of a Perpendicular Line.

Using the conditions from previous sections, we can find the equation of the perpendicular line to any given line’s equation, at a certain point.

Let, ax + by = c be a line, and we need to find a line perpendicular to it passing through

First, we will find the slope of the given line, 

Slope of a line m = -a/b

Now, using perpendicularity, if the slope of the second line is m’, then for these lines to be perpendicular

m × m’=- 1

⇒ m’ =- 1/m =- 1/-a/b = b/a

Thus, the slope of the perpendicular line is, 

m’= b/a

Then, we have a point as well as the slope for the equation of the perpendicular line,

Using point-slope form

If we know the exact values of a, b and then we can further simplify this equation.

Interesting Facts about Perpendicular Lines

• In order to obtain the maximum support for the roof, walls and pillars are constructed perpendicular to the ground in our homes and other buildings. This is just one example of how perpendiculars are used in everyday life.
• Perpendiculars of two lines that meet at an angle will also meet at that same angle.

Solved Examples

Example: Which of the following pair of lines are perpendicular, parallel, or simply intersecting?

1. Intersecting, since the angle of intersection here is given to be 100 degrees.
2. Perpendicular, as we know the right angle is also represented by a small square, we can say that these lines are perpendicular to each other.
3. Parallel lines, clearly extending these lines to infinity we will never see them intersecting; thus, they are parallel.
4. Perpendicular, clearly the angle of intersection here is given to be 90 degrees.

Summary

The subject of perpendiculars and the perpendicularity of lines were covered in this article. The reader should be able to comprehend the meaning of perpendicular lines, the symbol used to represent them, as well as the formula and theorems relating to perpendicular lines, after carefully reading this article. Two lines are said to be perpendicular if their angle of intersection is a right angle. The slopes of perpendicular lines are negative reciprocals of each other.

Frequently Asked Questions (FAQs)

1.What are Perpendicular Lines?

Ans. When two lines intersect at an angle of 90 degrees, the lines are said to be perpendicular to each other.

2. How do you Find the Slope of a line Perpendicular to a Given Line?

Ans. The slope of perpendicular lines are negative reciprocals of each other; thus, the slope of a perpendicular line can be found simply by negating the reciprocal of the slope of the given line.

3. What are Perpendicular Bisectors?

Ans. A line that divides another line segment into two halves while also being at a right angle to it is known as the perpendicular bisector of the line segment.

4. Are all Intersecting Lines Perpendicular?

Ans. No, all intersecting lines are not perpendicular, but all perpendicular lines are intersecting, that too at a specific angle, i.e., 90 degree.

Also read: Properties, Area of Right-Angled Triangles

Introduction

Pythagoras, a Greek philosopher who was born around 570 BC, is remembered by the theorem’s name. The theorem has likely been proved the most times of any mathematical theorem using a variety of techniques. The proofs are numerous, some of which go back thousands of years, and include both geometric and algebraic proofs. The Pythagorean theorem is extremely useful when determining the shortest distance between two points or the degree of the mountain slope. In a right-angle triangle the square of the hypotenuse is said to be equal to the sum of the squares of the two legs.

Right Angle Triangle

A triangle with a right angle is one in which one angle is 90 degrees. We refer to this triangle as a right-angle triangle since 90 is also referred to as the right angle. Triangle sides with a right angle were given unique names. The side directly opposite the right angle is known as the hypotenuse. Based on the values of the various sides, the right triangles are divided into isosceles and scalene types.

Properties of Right-Angle Triangle

• The height, base, and hypotenuse of a right-angle triangle are its three sides. 
• The two adjacent sides are referred to as base and height or perpendicular.
• Three similar right triangles are formed if we draw a perpendicular line from the vertex of a right angle to the hypotenuse.
• The radius of a circle whose circumference includes all three vertices is equal to one-half the length of the hypotenuse.
• The triangle is known as an isosceles right-angled triangle, where the adjacent sides to the 90° are equal in length if one of the angles is 90° and the other two angles are each equal to 45°.

Pythagoras Theorem

Pythagoras is a potent theorem that establishes the relationship between the sides of a right-angle triangle. According to Pythagoras’ theorem –

“Square of the hypotenuse is equal to the sum of the square of the other two legs of the right angle triangle”. Mathematically, it may be expressed as

              Hypotenuse² = Perpendicular² + Base² 

Area of the Right-Angle Triangle

The area of the right-angle triangle is the region enclosed within the triangle’s perimeter. The formula for a right-angle triangle’s area is.

Area of right-angle triangle = (Base × Perpendicular)

Facts

• A triangle must be a right triangle if it obeys Pythagoras’ theorem.
• The longest side of a triangle is the one that makes the largest angle.
• When the midpoint of the hypotenuse of a right-angled triangle is joined to the vertex of the right angle, the resulting line segment is half of the hypotenuse. In other words, the center of the hypotenuse is the circumcenter of the right-angled triangle.
• If two sides of a right angle are known, we can find the other side using Pythagoras’ Theorem.
• From the provided value of sides, we may determine whether a right-angle triangle is possible.

Summary

A right-angled triangle is one in which one of the angles is a right angle (90 degrees), and the hypotenuse is the side opposite to the right angle. The hypotenuse square of a right-angled triangle is equal to the sum of the squares of the other two sides, according to Pythagoras’ Theorem.

Solved Examples

Example 1: In the right-angle triangle, If PQ = 5 cm and QR = 12 cm, then what is the value of PR?

Solution:  By Pythagoras theorem, we have, 

   Hypotenuse² = Perpendicular² + Base²

PR² = 5² + 12²

PR² = 25 + 144

PR =  = 13 cm

Hence, the value of PR is 13 cm.

Example 2: If a triangle has three sides 9cm, 5 cm, and 7 am respectively, check whether the triangle is a right triangle or not.

Solution: According to the theorem, if the square of the longest side equals the sum of the squares of the other two sides, a triangle is said to be, a right triangle. 

9² = 5² + 7² 

81 = 25 + 49

81 ≠ 74

 Thus, 81 is not equal to 74. Hence, the given triangle is not a right-angle triangle.

Frequently Asked Questions

1.Which Side of a Right-Angled Triangle is the Longest?

Ans: The hypotenuse of a right-angled triangle is its longest side.

2.What is a Right-Angled Triangle’s Perimeter?

Ans: The perimeter of a triangle is the sum of all sides.

Perimeter = base + perpendicular + hypotenuse.

3.Can there be two Right Angles in a Triangle? Explain.

Ans: No, there can never be two right angles in a triangle. A triangle has exactly three sides and interior angles that add up to 180 degrees. This means that if a triangle contains two right angles, the third angle must be zero degrees, which means that the third side will overlap the opposite side. Therefore, a triangle with two right angles is not possible.

Introduction

Every day, new technological components are developed as technology advances throughout the globe. Electricity powers every other home, public space, and industry. People utilise electricity, and they use it for a variety of things. But how is it that this electric current has a particular level of power and continues to flow without any breaks? It is done with the aid of an object known as a conductor. Electric current may readily flow via the conductor. A conductor is built into anything that uses electricity to operate. These currents produce forces that flow in one direction. Let’s discover more about it.

Current Carrying Conductor 

A conductor that is transporting current can withstand the current’s force. Each current has a specific voltage that defines the electrical power. Electric bulbs can burst at high voltage, whereas low voltage results in weak electric current. There is no electric field surrounding the conductors. Unless a charge or electric field is given to it, it is neutral. The conductor’s sole responsibility is to transmit the current uninterruptedly to each source.

Magnetic Field due to Current Carrying Conductor.

A conductor that is conducting current generates a magnetic field everywhere around it. A current, as we all know, is a net charge that moves across a medium. The presence of moving charges in a conductor is a prerequisite for the creation of magnetic fields. Due to the magnetic fields‘ extra charge, an electric field is created. All of these elements help the current flow through a conductor smoothly.

Force on a Current Carrying Conductor in a Magnetic Field

A conductor experiences forces because of the external magnetic field. When two magnetic fields interact, there will be attraction and repulsion (according to their properties) based on the direction of the magnetic field and the direction of the current. That’s how a conductor experiences force. This phenomenon is termed Magnetic Lorentz force. This was found by H. A. Lorentz. This force is perpendicular to the direction of the charge and also to the direction of the magnetic field. It is a vector combination of the two forces.

The equation of the force on a conductor having a charge q and moving through a magnetic field strength of B is given as,

F = qvBsinθ

This equation can also be written as,

Where L is the length of the wire and t is the time. Rearranging the above equation, we get,

The Direction of a Force in a Magnetic Field

It is believed that the force acts perpendicular to the current’s direction. The left-hand rule is used to accomplish this. John Ambrose Fleming established this regulation. It is important to remember that the magnetic force is orthogonal to both the direction of motion and the charge velocity. Understanding which direction is applied to it is made easier by the left-hand rule.

State The Rule to Determine the Force or Direction

The direction of force, as we have seen in the article above, is perpendicular to both the magnetic field and the direction of the current. And the Right-hand rule-I decides this. The best mnemonic to remember the direction of force and current flow through the right hand is this example. The details are as follows:

• Place a hand between the magnetic field.
• The direction of the thumb points to the direction of the current (I).
• The fingers are facing the direction of the magnetic field (B).
• Now, the palm is facing the direction of the force (F).

Fleming’s Left-Hand Rule Definition

The current-carrying conductor will feel a force that is perpendicular to both the direction of the current and the magnetic field if it is put in the external magnetic field, according to a rule developed by John Ambrose Fleming. According to Fleming’s Left-Hand Rule, the thumb points in the direction of magnetic force, the forefinger points in the direction of the magnetic field, and the middle finger points in the direction of current if our forefinger, middle finger, and thumb are positioned perpendicular to one another. The late 19th century saw the development of this regulation.

Summary

Conductors have moving charges that are required for the magnetic field. Force moves in a perpendicular direction to the magnetic field and electric current. The magnetic field also exerts equal and opposite force in the current-carrying conductor.

Frequently Asked Questions

1. What is an Insulator?

Ans: We are aware that conductors enable uninterrupted electric current flow through them. However, it may also be prevented from flowing. Insulators carry out the work. Insulators are regarded as poor conductors of electricity because they do not permit electrons or atoms of materials to travel through them. Additionally, insulators have high resistance. Insulators still have some electric charge even if they prevent current passage. As a result, its primary use is high voltage resistance. Some examples are non-metals.

2. What are some High-Conduction Metals?

Ans: Metals that conduct heat and electricity in a very efficient way are called high-conduction metals, such that of gold, silver, and copper. In these materials copper is for construction purposes, making wires, cables, motors etc. because it’s cheaper than gold and silver. However, gold is used at very specific places due to its cost, and it is robust to environmental hazards like sulphur, oxygen, and water, whereas silver and copper react with environmental hazards.

3. What is a Semiconductor?

Ans: Semiconductors are materials that combine conductivity and insulator properties. Due to their capacity to both deliver and resist current flow, semiconductors are primarily employed in the production of electronic products and equipment. Doping the impurities into the crystal’s structure can change them. Silicon and gallium arsenide are two common semiconductors.