Tag: Linear

Introduction

Two lines can intersect at any point or come together at a common point to form an angle. An angle is defined as having two arms that extend outward, and its measurement is expressed in degrees. Angles in pairs are the related angles. Any pair of angles that have a specific relationship between them is therefore referred to as related angles. A specific name refers to these angles. Since the angles are related to a particular circumstance, they are known as related angles.

Related Angles

Related angles are those that have a particular relationship with one another. Each pair of connected angles is given a unique name. There is a specific standard for the associated angles.

Types of Related Angles

The related angles have specific names depending on the type of criteria. When the sum of the two angles is 90 degrees, they are said to be complementary angles. If the sum of the two angles is 180 degrees, they are said to be supplementary angles. In a plane, two angles are said to be adjacent if they share a common vertex, a common arm, and non-common arms that are on the opposite side of the common arm. Due to their shared arm, adjacent angles always lie next to their other pair. The two adjacent angles are regarded as a linear pair of angles when the sum of their respective measures equals 180 degrees. Since their non-common arms are two rays pointing in opposite directions, they are known as linear pairs of angles. When a transverse crosses parallel or non-parallel lines, various angles are created. Alternate exterior angles, alternate interior angles, vertically opposite angles, and corresponding angles are the different types of angles.

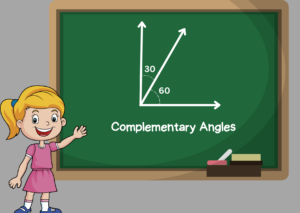
Complementary Angles

Complementary angles are those where the sum of the measures of two angles is 90 degrees.

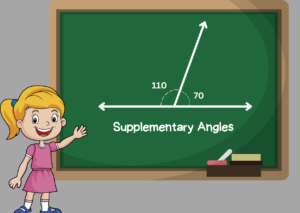
Supplementary Angles

These angles are referred to as supplementary angles when the sum of the measures of two angles is 180 degrees.

Adjacent Angles

If two angles in a plane share a vertex, a common arm, and their non-common arms are located on opposite sides of the common arm, then the angles are said to be adjacent.

Adjacent Angles: Linear Pair

If two adjacent angles share a common vertex, a common arm, and non-common arms that are oriented in opposition to one another, they are referred to as linear pairs of angles.

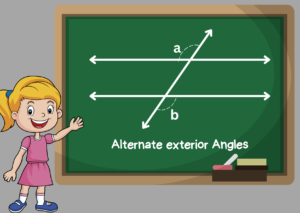
Alternate Exterior Angles

The angles outside the parallel lines and on the opposing sides of a transversal that intersects parallel lines are referred to as alternate exterior angles. Every other exterior angle is equal.

Alternate Interior Angles

The angles inside the parallel lines and on the opposing sides of a transversal that intersect parallel lines are referred to as alternate interior angles. All of the interior angles alternately are equal.

Vertically Opposite Angles

The angles are formed by two lines’ intersections, which are opposite. The angles that are vertically opposite are always equal in size.

Corresponding Angles

The angels that are lying parallel to the lines and on the same side of the transversal are always equal. Corresponding angles is the name given to these angles.

Interesting Facts about Related Angles

The linear angles’ and supplementary angles’ combined measures are equal. But the specifications for each of these angles vary. The placement of the angles is what causes this. A common arm will always connect the linear pair of angles. The supplementary angles, however, don’t always follow the same pattern. As a result, while all supplementary angles are not linear pairs, all linear pairs are supplementary angles.

Solved Examples

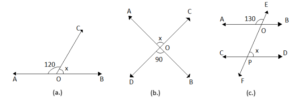
Example: Solve for x in the following images.

Solution: 

1.This is a linear pair that lies on line AB.

∠AOC + ∠COB = 180°

120° + x = 180°

x = 180 – 120

x = 60°

1.This is a pair of vertically opposite angles

x = 90°

1.Here, we have no direct connection between a given angle and the angle measured x.

Thus, we will use corresponding angles to find ∠CPO, to use the relation between ∠CPO and x.

∠CPO = ∠AOE

∠CPO = 130°

Now, ∠CPO and ∠OPD are a linear pair on the line CD.

∠CPO + ∠OPD = 180°

130 + x = 180

x = 180 – 130

x = 50°

Summary

There are specific requirements for the related angles. There are always two of them. The angles are referred to as supplementary angles if the total of the pair of angles is 180 degrees. The complementary angle pairs also add up to 90 degrees. The angles that are next to each other are those that have a common arm and other arms that are on different sides of the common arm. Adjacent angles make up the linear pair of angles. The linear pair of angles add up to 180 degrees.

Frequently Asked Questions (FAQs)

1.What are Related Angles?

Ans. Related angles are a pair of angles that have some sort of relation in their geometric structure, which gives them a relationship mathematically using a simple equation.

2.What is the Relationship between Angles on the Same Side of Transversal?

Ans. Angles on the same side of the transversal are shown below,

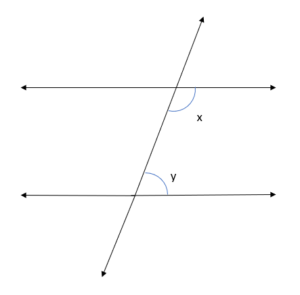
These angles are supplementary to each other, i.e., the sum of these two angles is 180 degrees.

In a pair of lines and a transversal, if corresponding angles are equal. What does it say about the pair of lines?

If in a pair of lines and a transversal, if the corresponding angles are equal, the pair of lines are parallel.

3.Are all Supplementary Angles Linear Pairs?

Ans. No, all supplementary angles are not linear pairs, since by definition linear pairs are the adjacent angles whose sum is 180 degrees, but for supplementary angles, the condition of adjacent angles need not be fulfilled.

Introduction

The relationship between two expressions on either side of the equal to sign is represented by an equation in mathematics. One equal symbol and one variable are used in this kind of equation. Simple equations use arithmetic operations to balance the expressions.

A simple equation is an equation that shows the relationship between two expressions on both sides of the sign. Only one variable appears in these kinds of equations, either on the first side or the other side of the equal symbol. For instance, 83 = 5 – 4z. In the provided example, the variable is z. Simple equations use arithmetic operations to balance the expressions on both sides. Linear equations in one variable are also regarded as simple equations.

Equations

Equations are relationships between two or more expressions connected by the equals sign, or “=.” Variables, coefficients, and constants are the three components of an equation.

Variables: Variables are the names given to the symbols (typically English alphabets) that are assigned to an arbitrary, unknowable value.

Coefficients: The coefficients of a term are the numbers that are multiplied by a variable or the product of two variables in that term.

Constants: Constants are the numbers that are independent of variables.

Simple Equations

A type of equation known as a simple equation compares two linear expressions with just one variable in common. Several instances of basic/simple equations are

3x + 4 = 7

4x + 5 = 3x + 8

Since many of the situations, we encounter in real life can be formulated as simple equation problems, we can use simple equations to obtain the desired results in a variety of areas of life.

Simple Equations questions

Simple equation problems, which can be represented by a simple equation to find the value of something unknown based on some given conditions, are known as simple equation questions. One such example of applying simple equations to real-world situations is provided, 

Let’s say Amar and Bipin, two friends, are purchasing apples. Amar might have purchased 5 kg and Bipin 3 kg. If Amar paid Rs. 80 more than Bipin, we must determine the cost of a kg of apples. The following simple equation can be used to represent this situation:

5x = 3x + 80, where x is the price of 1 kg apples.

Solving Simple Equations

To answer questions involving simple equations, we change the equation so that the term with the variables is on one side of the equation and the term with constants is on the other. We then simplify both sides so that there is only one term on each side, one with variables and the other with constants.

The value of the variable is then obtained by simply multiplying the equation by the reciprocal of the coefficient.

Now, let’s look at some examples to help us better understand it.

Example: Solve the following simple equation, 5x – 20 = 3x + 60

Solution: Here we have 5x – 20 = 3x + 60

Adding 20 to both sides while subtracting 3x to move terms with variables to one side and constants to the other.

⇒ 5x – 20 + 20 – 3x = 3x + 60 + 20 – 3x

⇒ 5x – 3x = 60 + 20

⇒ 2x = 80

Dividing by 2 on both sides

⇒ x = 40

Simple Equation Problems

Simple equation problems are mathematical issues from the real world that are modelled by simple equations. We must first determine the number of arbitrary values present and their relationships to represent a given situation using a simple equation. If there is only one arbitrary value, it is easy to create a simple equation to describe it; however, if there are several arbitrary values, we must establish a direct relationship between them to do so.

Example: Determine whether the following scenario can be modelled as a simple equation or not. Amit is currently twice as older than his younger brother Sagar. The combined age of Amit and Sagar was 23, two years ago. Identify their current ages.

Solution: Since Amit’s age and Sagar’s age are arbitrary values, the only way we can depict this situation in a simple equation is if there is a direct correlation between their ages, which is implied by the first statement that Amit is currently twice as old as Sagar. As a result, we can express this as a simple equation problem.

Let Sagar’s present age be x years

And Amit’s present age be y years

Then, ATQ

In present, y = 2x

Also, two years ago, (x – 2) + (y – 2) = 23

Substituting y = 2x in the second equation,

⇒ x – 2 + 2x – 2 = 23

Equations in Everyday Life Examples

When a value for a quantity or identity is unknown in a real-world situation and cannot be determined by a simple mathematical operation, linear equations are used, such as when estimating future income, forecasting future profits, or figuring out mileage rates.

Here are a few real-world instances where applications of linear equations are used.

• Can be used to identify age-related problems.
• It is used to determine the distance, duration, and speed of a moving object.
• It is used to resolve problems involving money, percentages, etc.

Solved Examples

Example: Calculate the value of y from the equation:  – 5 = 6.

Solution: We will simplify the equation first by separating the variables and constants,

– 5 = 6

Add 5 on both sides,

⇒  – 5 + 5 = 6 + 5

⇒  = 11

Multiply by 3 on both sides,

⇒  x 3 = 11 x 3

⇒ 11y = 33

Divide by 11 on both sides,

⇒ y = 3

Summary

Simple equations are also known as linear equations when they contain multiple variables and can be resolved using a variety of techniques. To solve problems from daily life, such as how to measure an unknown length, etc., we use simple equations. The typical method of representing the relationship between variables is through simple equations. A simple equation is a linear equation that only has one variable. Simple equations were credited to Rene Descartes as their creator. One of the foundations of algebra is simple equations.

Frequently Asked Questions (FAQs)

1. What are Linear Equations?

Linear equations are the mathematical relations that relate two expressions of degree 1 with the equal to symbol.

2. What are Simple Equations?

Simple equations are linear equations that have only one variable. Simple equations can be solved easily and are very useful in many days to day life problems.

3. What are the different methods of Solving Simple Equations?

There are two ways that we can solve simple equations. The techniques are the systematic method and the trial-and-error method.

4. What is a Rational Expression?

A rational expression is expressed in terms of the fraction of two algebraic expressions, and it also belongs to the class of simple equations.