Tag: Integers

Introduction

There are numerous quantities and measures that cannot be stated just in terms of integers. Rational numbers were crucial in expressing how such quantities were measured. These quantities included time, money, length, and weight. Some of the quantities for which the rational numbers are most frequently employed include those ones. Rational numbers are also required in trigonometry in addition to counting and measuring. The trigonometric ratios are expressed as rational numbers. Calculations based on the Pythagorean Theorem employ a specific kind of rational integer. 

Rational Number 

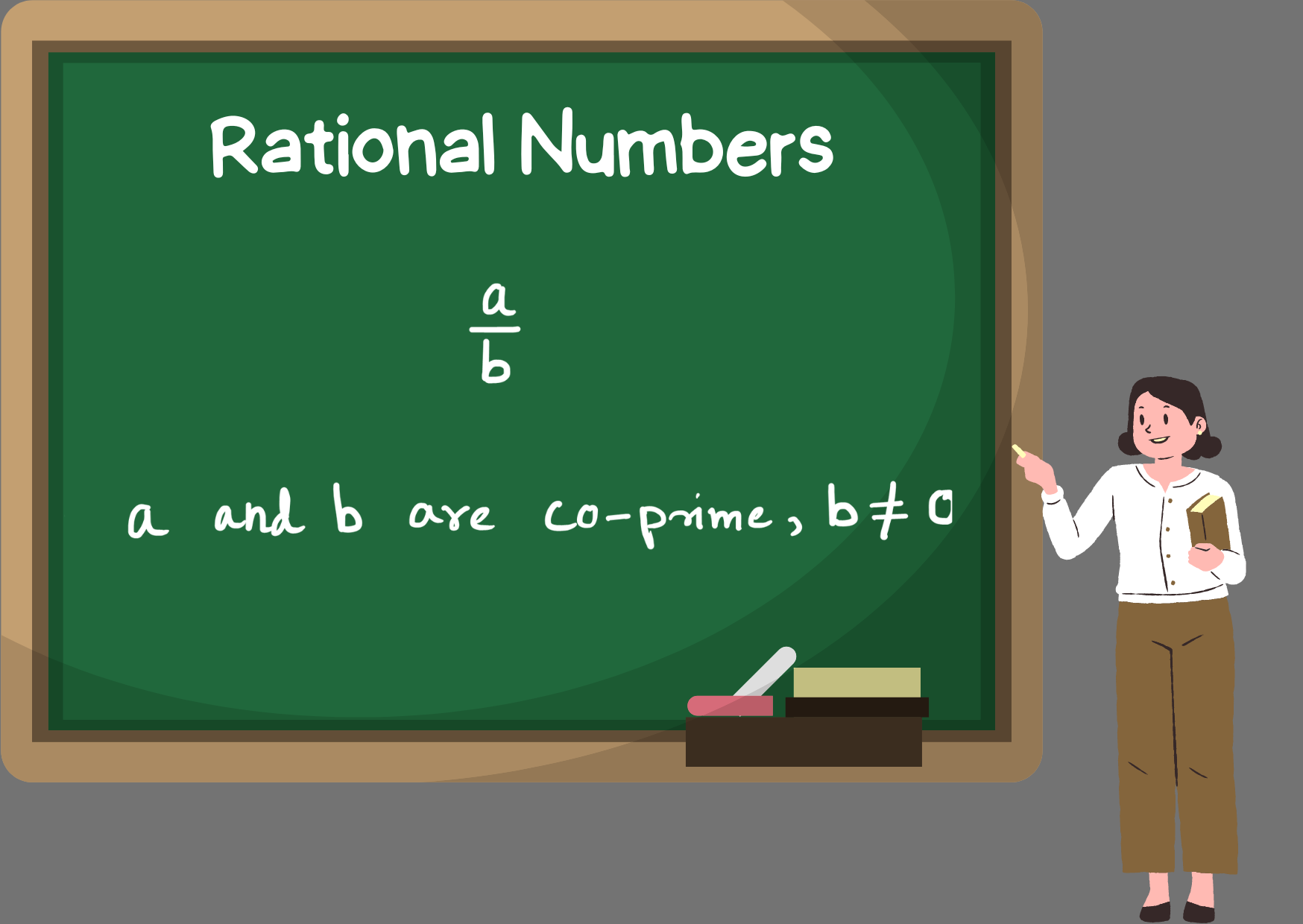
The rational number can be described as the ratios expressed in numbers. The term “rational” contains the word “ratio” as well. Therefore, any ratio is represented by rational numbers. These ratios may be lower than one to one or higher than one. Let’s comprehend how rational numbers should be explained. Rational numbers are defined as any number which can be expressed in the form of where a and b are coprime integers and b ≠ 0. The denominator is not equal to zero and both the numerator “a” and denominator “b” have integer values. The outcome of the division method used to simplify the rational number is in decimal form. The decimal representation of a rational number can either be non-terminating repeating decimals or terminating decimals.

How to find Rational Numbers

Verify that each given number meets the following requirements.

• The amount must be expressed as a fraction with a denominator greater than or equal to 0.
• To get the decimals, the fraction can be further decomposed.
• Positive, negative, and 0 are all included in the set of rational numbers, which is represented as a fraction. Because they may be written as a fraction, each whole number and integer is a rational number.

Types of Rational Numbers

Positive Rational Numbers

The positive rational numbers are signified as the rational numbers having positive numerators and denominators. The rational numbers  and are positive rational numbers.

Negative Rational Numbers

The negative rational numbers are signified as the rational numbers having any one of the numerators and denominators less than 0. The rational numbers  and are negative rational numbers.

Integers

The integers can be expressed as fractions having a denominator of one. Therefore, all integers are a class of rational numbers. Integers can have the forms of 0, -8, 56 etc.

You can also read our detailed article on Positive and Negative Rational Numbers.

Terminating Decimals

The decimals are the outcome of simplifying rational numbers. Some values following the decimal point may be where these decimals end. Terminating decimals are the name given to these rational numbers. For example: 0.235, 0.056, etc.

Non-Terminating Repeating Decimals are one Type of Rational Number.

Any rational integer is a non-terminating repeating decimal if, after simplification, the outcome is a decimal with repeating digits after the decimal point. A single digit or a group of digits can be one of the recurring values. For example: 0.5533, 0,222, 0.659659, etc.

Summary

Rational numbers are the numbers that can be written in the form of a fraction, where numerator and denominator are integers. The rational numbers are represented in the form of p/q where,q the denominator is not equal to 0.  Five separate categories of rational numbers exist. Both the numerator and the denominator are bigger than zero with positive rational numbers. Any numerator or denominator of a negative rational number is less than zero. Rational numbers that have a denominator of 1 are known as integers. The rational numbers also include recurring decimals that do not terminate.

Practice Solved Example

Example: The decimal expansions of some real numbers are given below. In each case, decide whether they are rational or not. If they are rational, write in the form of p/q. 

a. 0.140140014000140000…    

We have, 0.140140014000140000… It is a non-terminating and non-repeating. So, it is irrational. It cannot be written in the form of P/q.

b.

We have,    a non-terminating but repeating decimal expansion. So, it is Rational.

Let x =

Then, x = 0.1616 ——–1

100x = 16.1616 —-2

On subtracting 1 from 2 we get,

100x – x = 16.1616-0.1616

99x = 16

x =

Frequently Asked Questions

1.The number of Rational numbers between 25 and 26 is Finite. State the give statement is True or False.

Ans: False, any two rational numbers can be integrated by an infinite number of other rational numbers. Therefore, there are infinite rational numbers between 21 and 26.

2. Why does the Rational Number not have a 0 as its Denominator?

Ans: The outcome is not a defined value if the denominator of the rational number is 0. As a result, the rational number’s denominator never equals 0.

3. Can a Rational Number have a Numerator and Denominator of Zero?

Ans: No, the numerator may equal 0. However, for every rational number, the denominator can never be 0.

4. Which Technique is used to Transform a Rational number’s Standard form to Decimals?

Ans: The standard form of a rational number is converted to decimals using the division method.

Introduction

Natural numbers, their additive inverses, and zero are all collectively known as a set of Integers. We get a whole number when we subtract a small number from a larger number. However, there are no whole numbers that can represent the difference between a large number and a smaller number, such as 12 – 37. We created integers to describe such differences. Integer Addition follows all the rules of algebra, whereas Integer Subtraction doesn’t.

Addition and Subtraction of Integers

In addition, and subtraction of integers, we will learn how to add and subtract integers with the same and different signs. Certain rules must be followed when performing operations on integers.

When you add two positive integers, you get a positive integer, but when you add two negative integers, you get a negative integer sum. Adding two different signed integers, on the other hand, results in subtraction only, with the result having the same sign as the larger number. For example,

Addition with the same sign

3 + 5 = 8 or (- 7) + (- 5) =- 12

Addition with a different sign

6 + (- 4) = 2 or (- 6) + 4 =- 2

As for subtraction, when you subtract the integers, you add the additive inverse of the second integer, i.e., you can simply just change the sign of the second integer and add the two numbers using the rules of addition. For example,

7 – 5 = 7 + (- 5) = 2

9 – (- 2) = 9 + 2 = 11

(- 3) – (- 5) = (- 3) + 5 = 2

(- 6) – 7 = (- 6) + (- 7) =- 13

Properties of Integer Addition and Subtraction

Integers have a few properties that govern how they operate. These principles or properties can be used to solve a wide range of equations. Integers are any positive or negative numbers, including zero, to refresh your memory. These integers’ properties will aid in quickly simplifying and answering a series of integer operations.

All addition, subtraction, multiplication, and division properties and identities apply to all integers. The set of positive, zero, and negative numbers represented by the letter Z is known as the set of integers. Integers have the following five operational properties:

• Closure Property
• Commutative Property
• Associative Property
• Distributive Property
• Identity Property

Closure Property

According to the closure property, the set of all integers is closed under addition and multiplication, i.e., the addition or multiplication of any two integers will always result in an integer. Subtraction of integers also follows the closure property; division however does not follow the same rule for integers. For example, 2 divided by 5 is not an integer.

If a and b are two integers, then if,

c = a + b and d = a × b

Then both c and d are also integers

Changing b with – b,

e = a – b is also an integer.

Commutative Property

According to the commutative property of addition and multiplication, the order of terms does not affect the result. Let a and b be two integers, then by the commutative law:

a + b = b + a

Also,

a × b = b × a

But subtraction and division do not follow the same rules.

Associative Property

According to the associative property of addition and multiplication, it doesn’t matter how numbers are grouped; the result is the same. Regardless of the order of the terms, parenthesis can be used.

a + (b + c) = (a + b) + c

Also,

a × (b × c) = (a × b) × c

However, again, subtraction and division are not associative for Integers.

Distributive Property

The distributive property explains how one mathematical operation can distribute over another within a bracket. To make the calculations easier, the distributive property of addition or the distributive property of subtraction could be used. In this case, integers are multiplied or divided by each number in the bracket before being added or subtracted again.

a × (b ± c) = (a × b) ± (a × c)

Identity Property

When zero is added to any integer no matter the order, the result is the same number, according to the additive identity property of integers. Zero is known as additive identity.

Let a be an integer

Then, since 0 is known as the additive identity

a + 0 = a = 0 + a

Similar to this, we have a multiplicative identity. When a number is multiplied by 1 in any order, the product is the integer itself, according to the multiplicative identity property for integers.

Again, let a be an integer,

Then, since 1 is known as the multiplicative identity

a × 1 = a = 1 × a

Again, like most other properties, subtraction and division do not follow the identity property.

Solved Examples

Example 1: Simplify the following expressions

a. (- 2) + (- 4) – 5 + 13

b. 4 × 27 + 4 × 31 – 4 × 53

Solution:

a. (- 2) + (- 4) – 5 + 13

First, we will use parentheses to separate different operations

⇒ ((- 2) + (- 4) – 5) + 13

Here we have separated the only positive term, but we still have 3 terms in the parentheses,

⇒ (((- 2) + (- 4)) – 5) +13

Now solving the operations in the parentheses one by one,

⇒ ((- 6) – 5) + 13

⇒ (- 11) + 13

⇒ 2

b. 4 × 27 + 4 × 31 – 4 × 53

First, we can see that 4 is a common factor in all the products. So we will factor it out,

⇒4 × (27 + 31 – 53)

Now we will separate the terms inside the parentheses using centric parentheses

⇒ 4 × ((27 + 31) – 53)

No solving the parentheses one after the other

⇒ 4 × (58 – 53)

⇒ 4 × 5

⇒ 20

Summary

This article gives an insight into the properties of addition and subtraction of integers. According to the addition closure property, the sum of any two integers will always be an integer. According to the subtraction closure property, the sum of, or the difference between, any two integers will always be an integer. The commutative property of integer addition states that the order of addition of integers does not matter, the result remains the same regardless. Subtraction, on the other hand, is not commutative for integers. According to the associative property of addition, the order in which numbers are grouped does not affect the outcome. The nature of integer subtraction is not associative.

Frequently Asked Questions (FAQs)

1.What are Integers? How are they different from other types of Numbers, such as Natural Numbers and whole numbers?

Ans. Integers are numbers that have no decimal value, whole values represent them. The integers contain both positive and negative numbers, along with the number 0. The positive integers are known as natural numbers, whereas the positive integers with 0, aka the non-negative integers, are known as whole numbers.

2.What are the rules for Adding Integers?

Ans. The addition of integers follows two simple rules,

1. Adding two integers of the same sign results in the addition of the value of those numbers, and the result has the same sign as the additives.
2. Adding two integers of different signs results in a difference in the value of integers, and the result has the same sign as the number with the higher value.

3.Which Properties of Integer Addition can be applied to Integer Subtraction?

Ans. The following properties of integer addition can be applied to integer subtraction,

• Closure: The closure property states that the result of the operations is in the same set as the input, i.e., subtracting two integers will always result in an integer.
• Distributive: Distributive property states that the operation of multiplication can distribute over operations such as addition or subtraction, i.e., the product of a number and a difference of two numbers is the same as the difference of the product of the two numbers with the first.

4.What is an Inverse?

Ans. The inverse of a number is another number, which, when operated under a certain operation with the original number, results in the identity of that operation. That is, for addition, the inverse is defined as the number which when added together with the original number results in 0, and for multiplication, multiplies to give 1.

Introduction

Natural numbers, their additive inverses, and zero are all collectively known as a set of integers. We get a whole number when we subtract a small number from a larger number. However, there are no whole numbers that can represent the difference between a large number and a smaller number, such as 12 – 37. We created integers to describe such differences. Integer Addition follows all the rules of algebra, whereas integer subtraction doesn’t.

Division of Integers

We can use the properties of integer division because we are accustomed to working with whole numbers and natural numbers. 

The Division of Integers rules:

Rule 1: A positive integer is always the quotient of two positive integers or two negative integers.

Rule 2: A positive integer’s quotient when divided by a negative integer is always negative.

One important thing to keep in mind is that you should always divide without signs, but once you have the integer solution, give the sign following the sign specified in the problem.

Learn More about Properties of Division of Integers. Check out more videos in Maths Class 7 Lesson no 01.

Properties of Integer Operations

Integers have a few properties that govern how they operate. These principles or properties can be used to solve a wide range of equations. Integers are any positive or negative number, including zero, to refresh your memory. These integers’ properties will aid in quickly simplifying and answering a series of integer operations.

All addition, subtraction, multiplication, and division properties and identities apply to all integers. The set of positive, zero, and negative numbers represented by the letter Z is known as an integer. Integers have the following five operational properties:

• Closure Property
• Commutative Property
• Associative Property
• Distributive Property
• Identity Property

Closure Property

According to the closure property, the set of all integers is closed under addition and multiplication, i.e., addition or multiplication of any two integers will always result in an integer. Subtraction of integers also follows the closure property; division however does not follow the same rule for integers.

If a and b are two integers, then if,

c = a + b and d = a × b

Then both c and d are also integers.

But r = a/b is not always an integer.

Thus, the division of integers is not always closed.

Commutative Property

According to the commutative property of addition and multiplication, the order of terms does not affect the result. Let a and b be two integers, then by the commutative law:

a + b = b + a

Also,

a x b = b x a

But subtraction and Division do not follow the same rules.

Associative Property

According to the associative property of addition and multiplication, it doesn’t matter how numbers are grouped; the result is the same. Regardless of the order of the terms, parenthesis can be used.

a + (b + c) = (a + b) + c

Also,

a × (b × c) = (a × b) × c

However, again subtraction and Division are not associative for Integers.

Distributive Property

The distributive property explains how one mathematical operation can distribute over another within a bracket. To make the calculations easier, the distributive property of addition or the distributive property of subtraction could be used. In this case, integers are multiplied or divided by each number in the bracket before being added or subtracted again.

Multiplication is distributive from both sides, but Division is distributive only from the right side (denominator/divisor)

(a ± b) ÷ c = (a ÷ c) ± (b ÷ c)

Identity Property

When any integer is added to zero no matter the order, the result is the same number, according to the additive identity property of integers. Zero is known as additive identity.

Let a be an integer

Then, since 0 is known as additive identity

a + 0 = a = 0 + a

Similar to this, we have the multiplicative identity. When a number is multiplied by 1 in any order, the product is the integer itself, according to the multiplicative identity property for integers.

Again, let a be an integer,

Then, since 1 is known as the multiplicative identity

a × 1 = a = 1 × a

Again, like most other properties, subtraction and division do not follow the identity property.

BODMAS Rule

To simplify the calculations of more than two numbers having several types of operators, we have formed a rule that governs how to put parentheses around certain terms when solving the parentheses from the centre simplifies the calculation a lot.

This rule goes like

Bracket Of Division Multiplication Addition Subtraction – BODMAS

Here in this definition the ‘Of’ stands for functions such as exponents or square roots. So according to this rule, we put the centre of parentheses or brackets on subtraction, then addition, then multiplication and then division leaving the functions outside.

Solved Examples

Example: Are the following Integer operations closed (have an Integer result)?

a.  2 + 3/ (5 – 2)

b.  4(3/8) + 5/15

Solution:

a.  2 + 3/ (5 – 2)

Using BODMAS, we will simplify the terms by solving within the parentheses first.

⇒ 2 + 3/ (3)

⇒ 2 + 1 = 3

This operation is closed.

b.  4(3/8) + 5/15

Using BODMAS, we will simplify the terms by solving within the parentheses first.

This operation is not closed.

Also Read: Properties of Addition and Subtraction of Integers

Summary

This article discusses the topic of Integers, Integer Division. While also shining a light on the properties of Integers operations such as closure property, commutative property, etc. Integer division however does not follow most of these properties.

FAQs

 1. What are Integers? How are they different from other types of numbers, such as natural numbers and whole numbers?
Ans. Integers are numbers that have no decimal value, they are represented by whole values. The integers contain both positive and negative numbers along with the number 0. The positive integers are known as natural numbers, whereas the positive integers with 0, aka the non-negative integers, are known as whole numbers.

2. What are the rules for Dividing Integers?
Ans. The division of integers follows two simple rules,

1. Dividing two integers of the same sign results in the quotient of the value of those numbers and the result has a positive sign.
2. Dividing two integers of different signs results in the quotient of the value of integers and the result has a negative sign.

3. Which is the only Property of Integer Operations that Division follows, and on What Condition?
Ans. The distributive property is the only property of integer operations that the division of integers follows, and it follows the distributive property only from the right-hand side.