Tag: Fraction

Introduction

The most fundamental application of percentages is to compare two amounts while setting the second amount to 100. The use of percentages is widespread and varied aside from this. For instance, a lot of statistics in the media are expressed as percentages, including bank interest rates, retail discounts, and inflation rates. Percentages are essential for understanding the financial aspects of daily life.

The Latin word “per centum,” which means “per hundred,” is where the word “percent” originates. The task of comparing unlike fractions is very challenging. Because percentages are the numerators of fractions with a denominator of 100. Percentages have a wide range of applications in daily life, including making simple comparisons, allocating discounts in marketing, and calculating profits and losses for businesses.

Percentages

Per means “out of” in percentage, and cent means a century. In essence, the whole is always set to 100, and the relationship is between a part and the entire. The ratio known as a percentage has a denominator of 100 and the percentage symbol %.

The percentage formula is as follows:

How to find percentage from data?

To find percentage from a given data, we first need to find the fraction of the quantity from the given data. By dividing the number by a whole and multiplying the result by 100, any fraction can be expressed in percentages. Consider a society with 1000 members, 650 of whom are voters. Then the percentage of voters out of all the residents is,

% Of voters = × 100

= × 100

= 65%

Percentage of a Number

When a number is divided into 100 equal parts, the percentage of that number is the sum of those 100 parts. Calculating percentages involves multiplying the total number by the percent expressed as a fraction. For instance, let’s say we need to find 30% of 600. We can figure it out as:

30% of 600 = 600 ×  = 180

Here, 600 is total, so 30% of 600 is 180.

Applications of Percentages

One of the most useful mathematical ideas is the percentage, which has applications in practically every branch of science as well as in everyday life.

To compare fractions

Fractions can be converted to percentages to create a clear comparison representation. This is very useful when the denominators of two fractions have different values. For instance, suppose you need to compare the fractions 2/5 and 1/4. We use percentages because making a direct comparison in this situation is very difficult. For 2/5, the percentage form is 40%, and for 1/4, the percentage form is 25%. We can categorically state that 2/5 is greater than 1/4.

To estimate increment or decrement

Any change in a quantity can be expressed in terms of a percentage. For instance, a 20% decrease would occur if a person’s salary was Rs. 5000 per month one year and Rs. 4000 the following.

The formula to calculate % change in a quantity is

Change% = × 100

To calculate ‘How much’ or ‘How many’

Sometimes numbers are expressed as percentages, for example the case of a city where 40% of the people are vegan. Therefore, the percentage will enable us to determine the precise number of vegans.

The formula to calculate the number from percentage is as follows,

Quantity =  × Total

To calculate profit or loss in percentage, to mark discounts etc.

If the selling price and cost price are known, the seller can use percentages to determine its loss or profit. For instance, a seller may charge Rs. 5000 for a fan while only paying Rs. 4000. The profit margin in this case is 25%.

Solved Examples

Example: What is the discount given on an article marked Rs. 6000 with a discount of 30%.

Solution: The discount on the article is given by

Discount% = × 100

Discount = × Marked Price

Substituting values

Discount = × 6000 = Rs. 1800

A discount of Rs. 1800 is given.

Example: By what percentage is Amit’s salary, Rs. 35000, is less than from Sagar’s salary, Rs. 40000?

Solution: The difference between Amit’s and Sagar’s Salaries is

Sagar’s Salary – Amit’s Salary = 40000 – 35000 = Rs. 5000

The percentage difference between their salaries

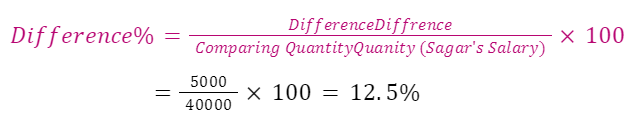
Thus, Amit’s salary is 12.5% less than Sagar’s salary.

Summary

When portions of a quantity are given, we have seen how to convert ratios into percentages. We discussed the formula for calculating the percentage of a number. An increase or decrease in a specified quantity can also be expressed as a percentage. The profit or loss in a specific transaction can be expressed in terms of percentages.

Frequently Asked Questions

1.What is the Importance of Percentage in Sciences other than Mathematics?

Ans. Percentage plays an important role in many aspects of business science, physical science, chemical science etc. In business science (statistics in particular) percentage is used to represent the parts of a data. In physical science percentage is used in various formulae, and derivations to represent efficiency, error and other such things. In chemical science percentage is used to calculate the concentration of different chemicals and composition of solutions etc.

2.How is Percentile Different from Percentage?

Ans. The value in the distribution or level at or below which a specific percentage of the score lies is represented by the percentile. For every hundred, which is the measurement unit, is referred to as the percentage.

3.What is the Relationship between Percentage and Probability?

Ans. Probability of an event can also be expressed as a percentage. For example, if the probability of an event is x, then the percentage probability is given by

% Probability = 𝑥 x 100%

Read More: Ratio and Percentage Formula and Examples

Introduction

Ratios are said to be equivalent if they can be made simpler or reduced to the same number. In other words, a ratio is said to be equivalent if it can be expressed as a multiple of another ratio.

A ratio can be expressed using a fraction. The concept of an equivalent ratio is comparable to the concept of equivalent fractions. The antecedent and consequent of a ratio can be multiplied or divided by the same number, other than zero, to create an equivalent ratio.

To get a ratio that is equal to the given ratio, we must first represent the ratio in fraction form. Then, by multiplying or dividing the first term and second term by the same non-zero value, the equivalent fraction can be found. We finally convert it to a ratio.

What are Equivalent Ratios

We must first comprehend what equivalence is to understand the equivalence of ratios. Equivalence is very similar to the well-known mathematical relation equal to, as well as to the same mathematical relationship between different objects. In mathematics, equivalence refers to the concept that two objects are equal but distinct because they have the same overall value. When two ratios share the same simplest form, they are said to be equivalent.

Examples of Equivalent Ratios

We can simply create equivalent ratios by multiplying the antecedent and consequent of a ratio by any real number other than the number zero. Thus, creating some examples of equivalent ratios is a very simple task.

For example, we need to find 5 ratios equivalent to 6:10

We can multiply the given ratio by any real number, let’s multiply it by ½

6: 10 = = 3: 5

Thus 3:5 is a ratio equivalent to 6:10

Other such ratios are, 9:15, 12:20, 15:25, 18:30, etc. these all are ratios equivalent to 6:10.

Methods of Finding Equivalent Ratios

There are two methods to find the equivalence of ratios, these methods are

• Cross Multiplication Method

In this method, we multiply the antecedent of the 1st ratio with the consequent of the 2nd ratio and the antecedent of the 2nd ratio with the consequent of the first. If the two products are equal then we can say that the two ratios are equivalent, otherwise, the ratios are not equivalent.

For example: Let’s say we need to use the cross-multiplication method to determine whether the ratios 3:4 and 6:8 are equivalent.

Therefore, we will multiply each ratio’s antecedent by the other ratio’s consequent.

We can say that the ratios are equivalent if the two products are equal.

In this example: 1st Product

3 × 8 = 24

2nd Product

6 × 4 = 24

Since,

Product 1 = Product 2

The ratios 3:4 and 6:8 are equivalent.

• HCF Method

In this method, we first need to represent the ratios as fractions, then we will reduce that fraction into standard form by finding the HCF of the numerator and the denominator, and then dividing the numerator and denominator by that HCF. After both fractions are reduced to the standard form, if they are equal, then the original fraction, i.e., the ratios were equivalent.

For example: Let’s say that we need to use the HCF method to determine whether the ratios 4:14 and 6:21 are equivalent.

To divide the antecedent and consequent of both ratios with their respective HCFs, we will first check the HCF of the antecedent and consequent for each ratio. If the two ratios are equal after the division, the original ratios were equivalent.

In this example: 1st ratio

4:14

HCF (4, 14) = 2

The ratio in the simplest form,

4: 14 == 2: 7

2nd ratio

6: 21

HCF (6, 21) = 3

The ratio in the simplest form

6: 21 = = 2: 7

Since both ratios in their simplest forms are 2:7, thus the original ratios were equivalent.

Use of Equivalent Ratios

In mathematics and other sciences, equivalent ratios have many applications. Some examples of uses are:

• To make the ratios provided simpler.
• We use equivalent ratios to solve any ratio-related problem.
• To calculate ratios between various fractions.
• Various direct proportionality-related scientific issues.

There are also a lot more uses like this.

Read: Applications of Percentage

Summary

We learned about the circumstances under which ratios or proportions are equivalent in this article. We discussed a few instances of equivalent ratios. The following ideas we learned were how to find equivalent ratios. We also discovered how ratios are equivalent using these techniques. Last but not least, we solved several cases that illustrated the concept of equivalent ratios.

Frequently Asked Questions

1. What are Ratios? What are the Components of Ratios?

Ans: Ratios are defined as a comparison between two quantities of the same type. A ratio has 3 parts, 2 parts are the numbers representing the compared quantities antecedent and consequent, and the third part is a symbol, specifically the ‘:’ (colon) symbol, that is put between the two to represent the comparison.

2. Why are Equivalent Ratios Important?

Ans: The equivalent ratios can be used to explain certain relationships between objects in daily life. For instance, if two pens cost Rs. 10, we can use equivalent ratios to determine the price of any other number of pens or the number of pens that can be purchased with a given sum of money. Many other real-world issues can be resolved using equivalent ratios.

3.What are Proportions? What is the Symbol of Proportions?

Ans: Proportions are a comparison between two or more ratios. If two ratios are in proportion, then they are also equivalent.