Tag: Article

Introduction

An expression made up of variables, constants, coefficients, and mathematical operations like addition, subtraction, etc. is known as an algebraic expression. Algebraic expressions have several uses, including describing real-world issues and solving various and difficult mathematical equations, calculating income, cost, etc. There are two categories of words in algebra: like terms and unlike terms. Unlike terms are merely the opposite of like terms in that they do not share the same variables and powers. Like terms are those that have the same variables and powers.

Algebraic Expressions

An expression made up of variables, constants, coefficients, and mathematical operations like addition, subtraction, etc. is known as an algebraic expression. A variable is a symbol without a predetermined value. A term is either a variable, a constant, or both joined by mathematical operations. A coefficient is a quantity that has been multiplied by a variable and is constant throughout the whole problem. Based on a variety of terminology, there are three primary categories of algebraic expressions: monomial, binomial, and polynomial. Terms can also be divided into similar and dissimilar terms.

Also see: Online Tuition for Class 6 Maths

Terms in algebra

A term can be a number, a variable, the sum of two or more variables, a number and a variable, or a product of both. A single term or a collection of terms can be used to create an algebraic expression. For instance, \({\bf{2x}}\) and \({\bf{5y}}\) are the two terms in the expression \({\bf{2x + 5y}}\) .

A mathematical expression has one or more terms. A term in an expression can be a constant, a variable, the product of two variables \({\bf{\left( {xy} \right)}}\) or more \({\bf{\left( {xyz} \right)}}\) , or the product of a variable and a constant \({\bf{\left( {2x} \right)}}\) , among other things.

Also Read: Terms of an Expression

Terms in an algebraic expression

A term is a group of numbers or variables that have been added, subtracted, divided, or multiplied together; a factor is a group of numbers or variables that have been multiplied; and a coefficient is a number that has been multiplied by a variable. Three terms, \({\bf{9}}{{\bf{x}}^2}\) ,\({\bf{x}}\)  , and \({\bf{12}}\), make up the expression \({\bf{9}}{{\bf{x}}^2}\)+\({\bf{x}}\) + \({\bf{12}}\)

By drawing this conclusion, it is clear that an expression is made up of a number of terms, variables, factors, coefficients, and constants.

Types of Terms 

There are different types of terms in algebraic expressions,

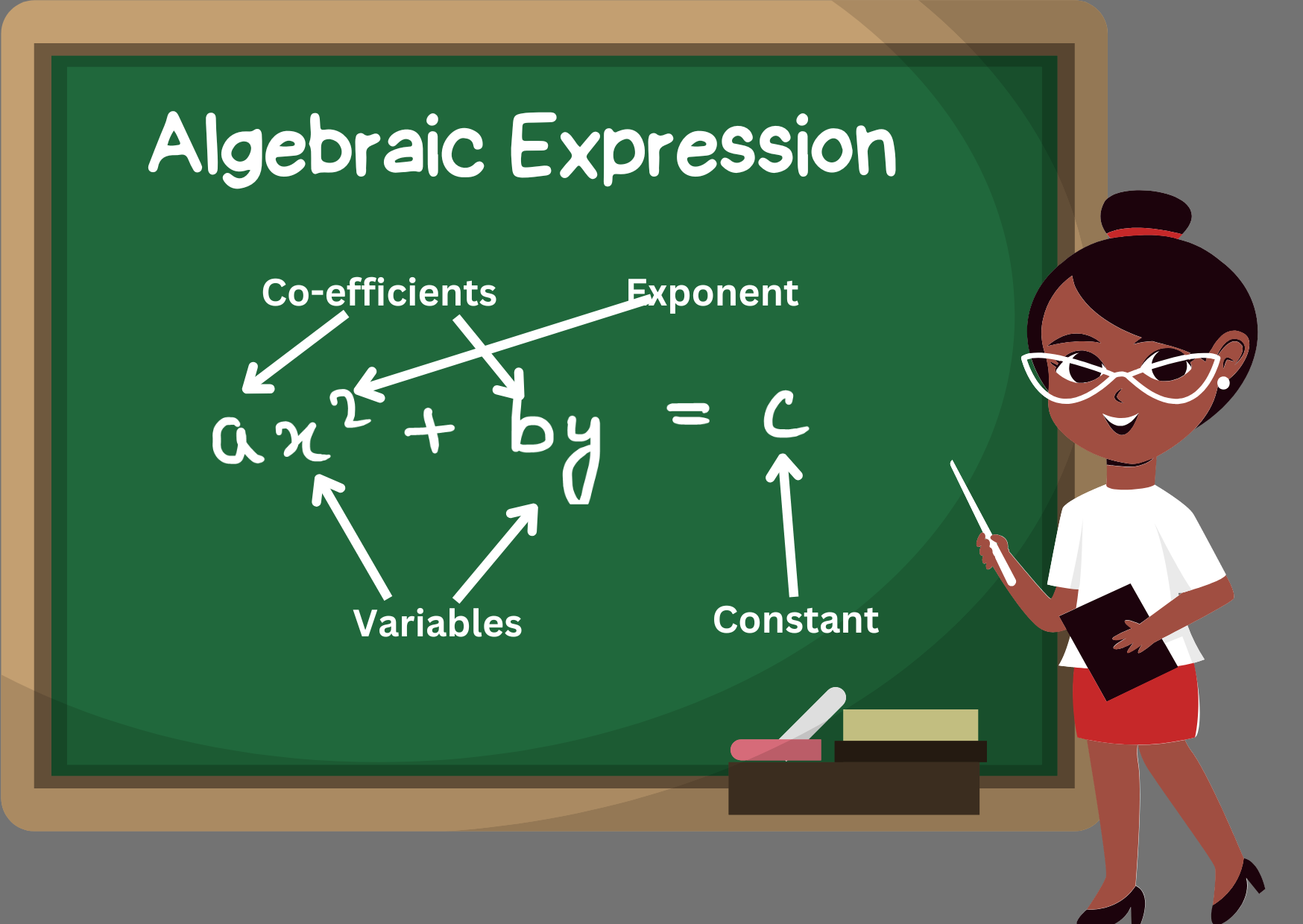
Variables

These types of terms are usually represented by an symbol (most commonly its english alphabets), like \({\bf{x}}\), \({\bf{y}}\), \({\bf{z}}\), \({\bf{a}}\), \({\bf{b}}\), etc. these symbols are there to represent unknown arbitrary values, hence the name ‘variables’ (since their values can vary).

Coefficient

These are not a type of a term but rather a part of a term that contains variables, coefficients are the numbers that are in multiplication with variables.

Constants

These terms are the numbers separate from the variables, and as the name suggest, they are a constant number, i.e., they are fix and never change unless they are under an operation with another constant term.

Like and Unlike Terms

Like terms in algebra are the kinds of terms that share the same kinds of variables and powers. There is no requirement that the coefficients match. When a term has two or more terms that are unlike terms, it means that those terms do not share the same variables or powers. Before there is power, the order of the variables doesn’t matter. Consider the example of similar and dissimilar terms.

Like Terms: \({\bf{3x}}\), \({\bf{-5x}}\) are like terms

Unlike Terms:\(\;{\bf{2}}{{\bf{x}}^3},{\bf{7}}{{\bf{x}}^2}\)  and \({\bf{5y}}\)  are all unlike terms.

Like Terms

Terms with the same kinds of variables and powers are referred to as like terms. It is not necessary to match the coefficients. The coefficient could differ. To obtain the answer, we can simply combine like terms, or we can simplify the algebraic expressions. In terms of the same types of variables and powers, the results are very easily obtained in this way.

The evaluation of straightforward algebraic puzzles is an example of similar terms.

Unlike Terms

In algebra, unlike terms are those terms that do not share the same variables and cannot be raised to the same power.

For instance, in algebraic expressions, \({\bf{4x}} – {\bf{3y}}\) are unlike terms. because \({\bf{x}}\) and \({\bf{y}}\) are two different variables. Due to the lack of \({\bf{x}}\) and \({\bf{y}}\) values, it cannot be simplified.

Summary

An expression made up of variables, constants, coefficients, and mathematical operations like addition, subtraction, etc. is known as an algebraic expression. Coefficients, constants, and variables are a few of the key words in the context of algebraic expressions. Similar terms are those in algebraic expressions that are constants or involve similar variables raised to similar exponents. In algebraic expressions, unlike terms are those terms that do not share the same variables or that share the same variables but have different exponents. Algebraic expressions are all polynomials, but not all algebraic expressions are polynomials. Polynomials are algebraic expressions without fractional or non-negative exponents. Algebraic expressions include fundamental identities that are used in the subject.

Tips to Prepare for Class 6 Maths

FAQs

What are polynomials?

Polynomials are algebraic expressions with more than \({\bf{2}}\) terms and the variables have non-negative integer exponents.

What is a quadratic equation?

A quadratic equation is a polynomial equation, with maximum exponent on a variable being \({\bf{2}}\).

What is a term in an algebraic expression?

A term can be a number, a variable, the sum of two or more variables, a number and a variable, or a product of both. A single term or a collection of terms can be used to create an algebraic expression.

Introduction

In geometry, an angle is made when two rays are joined at the same location. The common point is referred to as the node or vertex, while the two rays are known as the arms of the angle. We use the symbol “\(\angle \)” to represent an angle. The word “angle” has its roots in the Latin word “Angulus”, before talking about congruent angles, let’s define the term. In geometry, two figures are said to be congruent if their size and shapes are the same. That suggests that they will completely overlap if we stack one figure on top of the other. These figures may be line segments, polygons, angles, or 3D objects.

Congruent Angle Construction

Corresponding angles are always congruent on congruent figures. Two situations are there while learning about the construction of congruent angles in geometry. They are
• Any measurement can be used to create two congruent angles.
• making a new angle that is comparable to the one already there
Let’s look at two angles that are congruent with each other.

In the figure above, the angles are equal in size (\({100^\circ }\) each). They might completely encircle one another. As a result, both of the above angles satisfy the concept of congruent angles.

The symbol \(\angle 1 \cong \angle 2\) represents the congruence of two angles.

Angle Congruence Theorems

Many theorems are built on the concept of congruent angles. The congruent angles theorem allows us to easily assess whether two angles are congruent or not. The following are the theorems:

• The vertical angle theorem
• The corresponding-angles theorem
• The alternate-angles theorem
• Congruent supplement theorem
• Congruent complements theorem

Let’s talk about how these theorems are stated. Different theorems can be used to demonstrate if two or more angles are congruent. The following are the theorems:

The vertical opposite angle theorem

If two lines intersect each other, then the vertically opposite angles are equal.

Congruent Complements Theorem

The next theorem, the congruent complements theorem, states that if two angles are complements of the same angle, then the two angles are congruent.

Congruent Supplements Theorem

According to the Congruent Supplements Theorem, two angles are congruent if they are supplements of the same angle.

The alternate interior angles theorem

This theorem states that the alternate interior angles are equivalent if a transversal intersects two parallel lines.

The alternate exterior angles theorem

This theorem states that the angles formed on the outer side of the parallel lines and opposite sides of the transversal, when a transversal joins two parallel lines, have to be equivalent.

The theorem of Corresponding Angles

If a transversal meets two parallel lines, the corresponding angles that result are congruent.

Summary

This article showed us that a pair of angles cannot be congruent unless their measurements are equal. The statements of congruence of vertical angles theorem, corresponding angles theorem, alternate angles theorem, congruent supplements theorem, and congruent complements theorem were the next ideas we learned. We next discussed a few examples of congruent angles. We also learned how the angles are congruent based on these theorems. When two unknown angles are regarded as congruent, as well as how to calculate an angle’s measure, were both covered. Finally, we solved several cases that illustrated the concept of congruence of angles.

1. What is an angle? What are the measuring units of angles, and what is the relation between them?

Ans. An angle is the elevation of one line from another. Angles are measured in two main unit systems, i.e., the degree system and the radian system. The degree system has 2 sub-divisions, minutes and seconds. 1 complete circle is 360 degrees, 1 degree has 60 minutes and 1 minute has 60 seconds, the degree system angles are always represented by whole numbers and any fractional part is moved on to the next subdivision. Whereas the radian system has no subdivisions and can be represented in whole numbers, fractions and decimals. In the radian system, 1 complete circle is \(2\pi {\text{ rad}}\).

\[{360^\circ } = 2\pi {\text{ rad}}\]

\[{1^\circ } = \frac{\pi }{{180}}{\text{ rad}}\]

\[1{\text{ rad}} = {\frac{{180}}{\pi }^\circ }\]

2.What do you mean by congruent angles?

Ans. An angle is said to be congruent to another angle if the two angles are equal in measure.

3.Are the following two angles congruent?

Ans. Two angles are congruent if they are equal in measure, in the following image, the 1st angle, by the vertically opposite angles property, is a right angle. For, the second angle, using the corresponding angles property we can say that the angle adjacent to it is also a right angle. Now using the linear pairs,

\[{90^\circ } + \angle 2 = {180^\circ }\]

\[\angle 2 = {90^\circ }\]

Hence, the 2nd angle is also a right angle. Thus, the two angles are congruent.

Want to dive deeper into the topic of congruence? Check out our article on “Congruence for Triangles“

Introduction

The definition of a rational number is a fraction of two numbers in the form \(\frac{p}{q}\), where p and q can both be integers but q cannot be equal to 0. Rational numbers include whole numbers, integers, and numbers with terminating decimals. Although rational numbers need not necessarily be fractions, any fractions can be rational numbers. The area of mathematics that deals with symbols and variables are called algebra. Natural numbers, Integers, 0, and other types of numbers are all included in rational numbers. Positive and negative numbers are both part of integers. Therefore, we can divide rational numbers into positive rational numbers and negative rational numbers. Positive rational numbers include, for instance, \(1,\frac{3}{16},\frac{25}{2}\), etc. These are examples of negative rational numbers: \( – 3, – \frac{1}{2}, – \frac{5}{3}\), etc.

Rational Numbers

The definition of a rational number is a fraction of two numbers in the form \(\frac{p}{q}\), where p and q can both be integers but q cannot be equal to 0. Rational numbers include whole numbers, integers, and numbers with terminating decimals. Although rational numbers need not necessarily be fractions they can be converted into one, all fractions are rational numbers.

Positive and Negative Rational Numbers

Those rational numbers that have both positive or negative numerators and denominators are known as positive rational numbers. Positive numbers that follow logic are always bigger than zero. For instance, when we divide \(\frac{8}{9}\), we obtain 0.88, which is more than 0, indicating that \(\frac{8}{9}\) is a positive rational number.

Those rational numbers that are negative because their numerators and denominators have opposite signs are known as negative rational numbers. Positive irrational numbers are never greater than zero. For instance, \( – \frac{{12}}{{13}}\) yields -0.92, which is both lower than 0 and a negative rational integer.

Positive Numbers

The number line can also be used to represent positive numbers. According to the illustration below, the numbers that are on the right side of the number line are thought of as positive numbers. Positive numbers are those whose value is consistently higher than zero.

Note: When a number is left unsigned, it is considered to be positive. For instance, the positive integers 45 and +45 are identical and both 45.

Negative Numbers

Similar to the positive numbers, the number line can also be used to represent negative numbers. According to the illustration below, the numbers that are on the left side of the number line are thought of as negative numbers. Negative numbers are those whose value is consistently higher than zero.

Positive, 0 and negative rational numbers are the three subcategories of rational numbers.

A rational number is positive if both the numerator and the denominator have the same sign, such as both being positive or both being negative, and it is negative if the numerator is negative and the denominator is positive or vice versa.

Take an example of \(\frac{3}{5}\) and \(\frac{{ – 3}}{{ – 4}}\) they are both positive since the sign of both numerator and denominator are the same in the respective numbers, whereas \(\frac{{ – 3}}{4}\) and \(\frac{4}{{ – 5}}\) are both negative since the signs in numerator and denominator are opposite.

Algebra of Rational numbers

Rational Numbers like all the other number categories under the real number have four basic binary operations, i.e, addition, multiplication and the inverse operations subtraction and division.

Addition and Multiplication of Rational numbers have the following properties,

Closure Property

Addition and Multiplication of rational numbers is closed, i.e., when two rational numbers are operated with these operations the result is always rational number.

Associative Property

Addition and Multiplication of rational numbers is associative, i.e., the order of operations does not change the result when the same operation is repeated between 3 or more numbers. Mathematically, for three rational numbers a, b and c

\[a + (b + c) = (a + b) + c{\text{ and }}a \cdot (b \cdot c) = (a \cdot b) \cdot c\]

Existence of Identity Property

The Identity element is known as the element which when operated with any other element, has no effect on it. For Addition of rational numbers, 0 is the identity element, and for multiplication it is 1.

Mathematically,

\[a + 0 = 0 + a = a{\text{ and }}a \cdot 1 = 1 \cdot a = a\]

Existence of Inverse Property

The Inverse of an element is known as the element which when operated with the first element, results in the identity. Rational numbers have additive inverse for all the numbers, and they are their negative counterparts, such as for 3 it is -3, for \(-\frac{5}{6}\) it is \(\frac{5}{6}\). For multiplication however, not all rational numbers have inverse, 0 is the rational number which does not have a multiplicative inverse, because by definition multiplicative inverse of a rational number \(a\) would be \(\frac{1}{a}\), but by the definition of rational numbers, 10 is not a rational number.

Commutative Property

Addition and Multiplication of rational numbers is associative, i.e., the order of element does not change the result. Mathematically, for two rational numbers a and b,

\[a + b = b + a{\text{ and }}a \cdot b = b \cdot a\]

Summary

In this article we learned about Rational numbers, positive and negative rational numbers. A rational number is defined as the number which can be represented by the form, \(\frac{p}{q}\) where p and q are coprime integers and \(q\ne 0\). The rational numbers, just like integers, can be divided into 3 categories i.e., positive, negative and 0. Positive rational numbers are those that are greater than 0, and negative are the ones that are smaller. The positive and negative rational numbers are represented on right and left sides of the number line respectively.

FAQs

What are Rational numbers? How do you identify rational numbers in decimal form?

Ans. Rational numbers are defined as the numbers which can be represented by the form, \(\frac{p}{q}\) where p and q are coprime integers and \(q \ne 0\). In decimal form, the numbers that have either terminating decimal expansion or if non-terminating then, repeating decimal expansion are rational numbers.

What is Rule for identifying positive and negative rational numbers from their fractional form?

Ans. There is one simple rule to identifying positive and negative rational numbers from fractional form

• If the numerator and denominator have the same sign, both either positive or negative, then the number as a whole is positive.
• If the numerator and denominator have different signs, numerator positive and denominator negative or vice versa, then the number as a whole is negative.

What are the numbers that are not rational called? What is the general identification of those numbers in the decimal form?

Ans. The real numbers that are not rationals, i.e., they cannot be represented in the form, \(\frac{p}{q}\) where p and q are coprime integers and \(q \ne 0\), are known as Irrational numbers. These are the numbers whose decimal expansion has infinite digits after the decimal, and they never repeat the same pattern.

Introduction

Only when two figures have the same size and shape, including their sides, points, angles, etc., can they be said to be congruent. 

1. Two circles should have the same diameter if they are congruent. 
2. If the sides and angles of two triangles are the same, they are said to be congruent. 
3. If the corresponding sides of two rectangles are equal, they are said to be congruent. 
4. If two squares have sides of the same length, they are said to be congruent.

If two shapes are equivalent to one another in all conceivable ways, they are said to be congruent. Congruent figures in mathematics are those that share the same size and shape. The 2-D and 3-D figures are both consistent with each other. However, this article will only discuss the congruence of plane figures. 

Figures that are consistent in size and shape are said to be congruent. Congruence is the name given to the relationship between two congruent figures. It is indicated by the symbol “≅“.

Plane Figures

A plane shape is a closed, 2-D, or flat figure. Different plane shapes have various characteristics, such as various vertices. A vertex is the point where two sides meet, and a side is a straight line that is part of the shape.

The following figures show some of the basic plane shapes: triangles, squares, rectangles, and circles.

Congruence of Plane figures

A geometric figure with no thickness is called a plane figure. Some of the plane figures include line segments, curves, or a combination of both line segments and curves. The sides of the plane figures are the straight lines or curves  that make them up.

If two plane figures, such as line segments, angles, and other figures, are similar in size and shape, they are said to be congruent. Congruence of plane figures is the name of the relationship in use.

Congruent Figures

Congruent figures are geometric objects that share the same size and shape in mathematics. The two figures are equal to one another and are referred to as congruent figures when you transform one figure into another by a series of rotations and/or reflections.

Congruence of Lines

If two line segments are of the same length, they are said to be congruent. They don’t have to be parallel, though. They are flexible and can be in any position or orientation. The separation between two points determines the length of a line segment.

Congruent Line Segments

A pair of equal-length line segments makes up the congruent segment. An exact starting point and ending point define a straight line segment. Its beginning and end points are known, so its length can be calculated. Congruent line segments can, but are not required to, be parallel, perpendicular, or at any other particular angle. 

In geometry, a line segment is a fundamental figure that is created by joining any two points on a plane figure. Line segments also make up the sides of the plane figures. Two line segments are said to be congruent if their lengths are the same. In other words, two line segments have equal lengths if they are congruent.

Rules of Congruence for Triangles

There are 5 basic rules of congruence:-

Side Side Side

Side Side Side or also known as SSS congruence criteria states that if  the three sides of one triangle are equal to the corresponding three sides of another triangle, then the triangles are congruent.

Side Angle Side

Side Angle Side or also known as SAS congruence criteria states that if two sides and the included angle of one triangle  are equal to the corresponding two sides and the included angle of another triangle , then the triangles are congruent.

Angle Side Angle

Angle Side Angle or also known as ASA congruence criteria states that if  two angles and the included side of one triangle are equal to the corresponding two angles and the included side of the another triangle,  then the two triangles are congruent.

Angle Angle Side

Angle Angle Side or also known as AAS congruence criteria states that if two angles and a side not common to the two angles of one triangle are equal to the corresponding angles and side of another triangle, then the triangles are congruent.

Right angle Hypotenuse Side

Right angle, Hypotenuse & Side or RHS congruence criteria only applies to right triangles, it says that in two right triangles if  the hypotenuse and one  side of a triangle are equal to the hypotenuse and the corresponding side of the other triangle, , then the triangles are congruent.

Summary

In this article the topic of congruence is discussed in detail. If two figures share the same shape and size, they are said to be congruent; alternatively,  a figure  is said to be congruent to its mirror image as they share the same shape and size. Figures drawn on a plane or other flat surface are referred to as “plane figures.” In geometry, a plane is a flat surface that can go on forever in all directions. It has infinite width and length, no thickness, and curvature as it is stretched to infinity.

This article also shines a light on the topic of Rules of Congruence for triangles. There are 5 basic congruence criteria.

Frequently Asked Questions

1. What do you mean by congruence?
   Ans. Congruent figures are geometric objects that share the same size and shape in mathematics. The two figures are equal to one another and are referred to as congruent figures.
   
2. Are all squares congruent?
   Ans. No, all squares are not congruent, since for congruence two figures must have all of their quantifying dimensions  equal, that includes all the sides and all the angles. All squares have the same angles, but their side lengths may be  different, hence they aren’t congruent.
   
3. Is AAA a criteria for congruence of triangles?
   Ans. No, AAA is not a criteria for congruence because even if all the angles of two triangles are correspondingly equal, that necessarily does not mean that they have the same side length, for example two equilateral triangles of sides 3cm and 5cm, they both have the same 60-60-60 angle but they are not congruent because their sides are of different lengths.
   
4. Is a Rhombus of side length 4cm congruent to a square with side 4cm?
   Ans. We know that both rhombus and square have the property that all their sides are of the same length. But a rhombus does not necessarily have the same angles, whereas by definition a square has all its angles 90 degrees. Hence, no, a rhombus of side length 4cm is not necessarily congruent to a square of side length 4cm.

   Also Read: Congruence of Angles

Introduction

A straight line that intersects another straight line at a 90-degree angle is said to be perpendicular to the first line. The small square in the middle of two perpendicular lines in the figure represents 90 degrees, also known as a right angle. Here, two lines cross at a right angle, indicating that they are perpendicular to one another.

In contrast to sloping or horizontal lines or surfaces, perpendicular lines or surfaces point directly upward. An object is at a 90-degree angle to another if it is perpendicular to it. A pair of lines, vectors, planes, or other objects are said to be perpendicular if they intersect at a right angle. Two vectors are perpendicular if their dot product equals zero.

Perpendiculars

When two lines intersect at a right angle, they are said to be perpendicular to one another. A first line is perpendicular to a second line, more specifically, if the two lines intersect and the straight angle on one side of the first line is split into two congruent angles by the second line at the intersection. Since perpendicularity is symmetric, if one line is perpendicular to another, the other line is also perpendicular to the first. As a result, we don’t need to specify an order when referring to two lines as perpendicular (to one another).

Perpendicular Lines

Segments and rays are easily extended by perpendicularity. If a line segment AB and a line segment CD result in an infinite line when both directions are extended, then the two resulting lines are perpendicular in the sense mentioned above. Line segment AB is perpendicular to line segment CD and can be represented by the symbol AB ⊥ CD. If a line crosses every other line in a plane, it is said to be perpendicular to the plane. The definition of line perpendicularity is necessary for understanding this definition.

Perpendicular Theorem

According to the perpendicular line theorem, two straight lines are perpendicular to one another if they intersect at a point and create a pair of equal linear angles.

Assume two lines AB and CD intersect each other at O, such that ∠AOC = ∠COB, also since AB is a line, ∠AOC and ∠COB also form a linear pair.

Then, ∠AOC + ∠COB = 180°

Using ∠COB = ∠AOC

⇒ ∠AOC + ∠AOC = 180°

⇒ 2 ∠AOC = 180°

⇒ ∠AOC = 90°

Thus, since the angle of intersection is 90°, we can say that AB is perpendicular to CD and vice versa.

Also Read: Related Angles

Perpendicularity: Slope Formula

Perpendicularity is known as the mathematical condition that two lines need to satisfy to be called perpendicular. Mathematically, if two lines are perpendicular to each other, then the product of their slopes is negative unity.

For example, let two lines of slope . Then these lines are said to be perpendiculars to one another if their slopes have a product -1, i.e.,  

Equation of a Perpendicular Line.

Using the conditions from previous sections, we can find the equation of the perpendicular line to any given line’s equation, at a certain point.

Let, ax + by = c be a line, and we need to find a line perpendicular to it passing through

First, we will find the slope of the given line, 

Slope of a line m = -a/b

Now, using perpendicularity, if the slope of the second line is m’, then for these lines to be perpendicular

m × m’=- 1

⇒ m’ =- 1/m =- 1/-a/b = b/a

Thus, the slope of the perpendicular line is, 

m’= b/a

Then, we have a point as well as the slope for the equation of the perpendicular line,

Using point-slope form

If we know the exact values of a, b and then we can further simplify this equation.

Interesting Facts about Perpendicular Lines

• In order to obtain the maximum support for the roof, walls and pillars are constructed perpendicular to the ground in our homes and other buildings. This is just one example of how perpendiculars are used in everyday life.
• Perpendiculars of two lines that meet at an angle will also meet at that same angle.

Solved Examples

Example: Which of the following pair of lines are perpendicular, parallel, or simply intersecting?

1. Intersecting, since the angle of intersection here is given to be 100 degrees.
2. Perpendicular, as we know the right angle is also represented by a small square, we can say that these lines are perpendicular to each other.
3. Parallel lines, clearly extending these lines to infinity we will never see them intersecting; thus, they are parallel.
4. Perpendicular, clearly the angle of intersection here is given to be 90 degrees.

Summary

The subject of perpendiculars and the perpendicularity of lines were covered in this article. The reader should be able to comprehend the meaning of perpendicular lines, the symbol used to represent them, as well as the formula and theorems relating to perpendicular lines, after carefully reading this article. Two lines are said to be perpendicular if their angle of intersection is a right angle. The slopes of perpendicular lines are negative reciprocals of each other.

Frequently Asked Questions (FAQs)

1.What are Perpendicular Lines?

Ans. When two lines intersect at an angle of 90 degrees, the lines are said to be perpendicular to each other.

2. How do you Find the Slope of a line Perpendicular to a Given Line?

Ans. The slope of perpendicular lines are negative reciprocals of each other; thus, the slope of a perpendicular line can be found simply by negating the reciprocal of the slope of the given line.

3. What are Perpendicular Bisectors?

Ans. A line that divides another line segment into two halves while also being at a right angle to it is known as the perpendicular bisector of the line segment.

4. Are all Intersecting Lines Perpendicular?

Ans. No, all intersecting lines are not perpendicular, but all perpendicular lines are intersecting, that too at a specific angle, i.e., 90 degree.

Also read: Properties, Area of Right-Angled Triangles

Introduction

The Khalji dynasty reigned during the height of the Delhi sultanate, and Allaudin Khalji was credited with most of its accomplishments. He united the entire Indian subcontinent under his reign and was the most powerful sultan of the Delhi sultanate. After slaying his father-in-law Jalaluddin Khalji, the founder of the dynasty, Alauddin Khalji ascended to the throne in 1296. In 1320, the Ghiiyasuddin Tughluq overthrew the Khalji dynasty and established the Tughlaq dynasty. Later, Mohammad Tughluq oversaw the growth of the Sultanate.

Consolidation under Khalji Dynasty

Due to internal strife among the noles, Jalaluddin, the first king of the Khalji dynasty, was unable to extend his territory. He had a brief six-year reign during which he spent time stabilising and legitimising his position and power. Alauddin Khalji, Jalaluddin’s son-in-law, killed him, took the throne for himself, and proclaimed himself the ruler of the Delhi sultanate.

The Delhi Sultanate reached its pinnacle under Alauddin Khalji. Alauddin’s first trip was to Gujrat in 1299. This was his first foray into new territory. He stole the wealth and appointed Alp Khan governor. He continued his westward expansion by attacking Malwa in 1305 and winning a bloody struggle to take the fort of Mandu. He seized control of Chittor, Mewar, and Ranthambore as well as all of western India. Alauddin extended his hegemony into southern India. Wherever he triumphed, he appointed his trusted nobles as administrators.

Administration under the Khalji Dynasty:

The kings of the Khalji people appointed their military leaders as governors and gave them authority over their territory. These territories were referred to as “Iqta” and the owners as “Iqtadar” or “Muqti.” Iqtadars were required to support the king militarily and uphold law and order in their region. Iqtadar received a wage from the money their territories generated. Three different tax types existed. The first tax was imposed on the “kharaj” portion of the crop, followed by a tax on cattle and a third tax on horses. Throughout Alauddin Khalji’s reign, numerous administrative changes were made.

• The empire was divided into provinces, and there were 11 provinces under Alauddin Khan.
• Alauddin established a sizable standing army to defend the country from Mongol intrusion.
• For his troops, Alauddin built the garrison town of Siri.
• He also levied taxes in the Ganga Yamuna doab region to pay for the rations of his soldiers. He set the prices for goods in Delhi; government employees were assigned to monitor this, and those who failed to sell at the set price faced consequences.
• The first emperor to pay his troops in cash was Alauddin. Alauddin had managed the market price so that it stayed constant even during the Mongol invasion.

Consolidation under Tughlaq Dynasty:

The Deccan Sultanate’s rule was not unbreakable; with the death of Alauddin, the southern provinces rose up and gained their independence. The founder of the Tughlaq dynasty, Ghyisuddin Tughluq, was made aware of this. During the brief period of his administration, Ghiyasuddin was unable to subjugate the south to the Delhi sultanate. After assuming power, Mohammad bin Tughluq concentrated his effort on the south. He organised numerous military operations and seized control of a sizable portion of the South.

He went on to Mabar in the south. He conquered Bengal in the east, which had declared itself independent because of its separation from the Delhi sultanate and the difficulty of maintaining administration and consolidation at such a distance.

Mohammad Tughluq organised a number of far north and northwest missions. After suffering a severe defeat in Tibet with his troops, he planned the Qurachi expedition but later abandoned it. The Delhi Sultanate’s largest domain belonged to Mohammad Tughluq, and this contributed to the sultanate’s demise.

Administration under Tughlaq Dynasty:

The Tughlaq dynasty preserved the empire while carrying out the majority of Khalji’s administrative principles. Nobles were given the authority to collect taxes from their iqta as part of the tradition of iqta. Bandagan continued to be appointed as governor and military commander under Tughlaqs. Specially trained slaves called bandagan were loyal only to the King. Some rules imposed by the king were highly controversial. He appointed gardeners, cooks, and wine distillers to high administrative posts at once. The noles were harshly critical of the ruler’s unorthodox methods.

• To stop the Mongols from capturing their empire, Mohammad established a powerful standing army.
• His victory over the Mongol invasion. Instead of building a new garrison town for the army, he dispersed its population to Daulatabad and stationed his soldiers in an old Delhi neighbourhood.
• Taxes were raised to provide for the troops.
• People started to feel unsatisfied as a result.
• At the same period, north India had a famine.
• His attempt at token currency, which was made of cheap metal and was simple to reproduce, failed horribly.
• Taxes were paid with token money, and gold was kept.

Summary

The Khalji and Tughlaq dynasties represented the height of the Delhi sultanate. The Khalji monarch Alauddin implemented strict laws and regulations to manage his enormous realm. He was an astute administrator who worked hard to keep Delhi’s commodity prices stable. The Tughlaq dynasty inherited Khalji’s administrative and expansionist objectives. Mohammad Tuhghluq, the most well-known king of Tughlaq, introduced many radically new administrative reforms and conducted numerous policy experiments. Unfortunately, he had short-sighted policies and was a hasty and irritable ruler, which led to the slow decline of his kingdom.

Frequently Asked Questions

1.What were Muqtis’ Responsibilities?
Ans. Muqties were required to uphold peace and order in their iqtas as well as provide the emperor with military support. Muqties were permitted to deduct taxes from his iqta in exchange for their services.

2.Who were the Chieftains?
Ans. Chieftains were another name for Samanta nobility. They were wealthy landowners who lived in the countryside, and the empire brought them under its control and taxed them.

3.What is Accurate in terms of Governance and Unification under the Khaljis and Tughlaqs?
Ans. Even though these dynasties ruled over the majority of the Indian subcontinent, most of the interior remained independent. The hardest part of managing all the provinces was the distance, and remote areas like Bengal were tough to manage.