Cyclic Quadrilateral

Introduction

A quadrilateral that can be completely inscribed in a circle is called a cyclic or inscribed quadrilateral and conversely, a circle passing through all four vertices of a quadrilateral is known as a circumcircle. The centre of such a circle is called the circumcentre and the radius is known as the circumradius. Another way of saying that a quadrilateral is cyclic is to say that its vertices are concyclic. Interestingly, while you can inscribe all triangles into a circle, the same is not possible with all quadrilaterals and instead, only some of them can be cyclic.

Definition – What is cyclic quadrilateral

A quadrilateral with all its vertices lying on a circle is called a cyclic quadrilateral. However, not every quadrilateral can be inscribed in a circle and thus, the quadrilateral must be cyclic by design.

The figure below shows a cyclic quadrilateral EFGH inscribed inside a circle. Its sides are represented by e, f, g, and h, and the diagonals are represented by p and q. Note that the diagonals need not be of equal length.

A cyclic quadrilateral

Angles

Angles opposite to each other inside a cyclic quadrilateral sum up to 180⁰, i.e., they are supplementary. For instance, in the figure shown above, we have a cyclic quadrilateral EFGH. If the angles made at the vertices of this quadrilateral are represented by ∠E, ∠F, ∠G, and ∠H, respectively, then we can write the following:

\(\angle E + \angle G = {180^0}\)

\(\angle F + \angle H = {180^0}\)

Further, just like all other quadrilaterals, the sum of all the angles of a quadrilateral is equal to 360⁰ and this can be easily proven by adding the two equations written above.

Radius

There are a few other interesting properties related to the side lengths of a cyclic quadrilateral. Given the cyclic quadrilateral EFGH as above, we can write the following:

semi perimeter of circumcircle, s = \(\frac{{e + f + g + h}}{2}\)

Radius of circumcircle\(r = \frac{1}{4} \times \sqrt {\frac{{(eg + fh) \times (eg + fh) \times (eh + fg)}}{{(s – e) \times (s – f) \times (s – g) \times (s – h)}}} \)

Diagonals

Once again, we look at the cyclic quadrilateral we saw above, with diagonals represented by p and q. Another interesting property that emerges is between the side lengths and diagonals of such a quadrilateral. We can write the following:

length of diagonal p \( = \frac{{(eg + fh) + (eh + fg)}}{{(ef + gh)}}\)

length of diagonal q \( = \frac{{(eg + fh) + (ef + gh)}}{{(eh + fg)}}\)

Area

We can also examine properties related to the area of cyclic quadrilaterals. Looking at the figure shown before, if we have the semi perimeter given by s, we can write the following:

semi perimeter s \( = \frac{{e + f + g + h}}{2}\)

Area \( = \sqrt {(s – e) \times (s – f) \times (s – g) \times (s – h)} \)

Theorems

Ptolemy’s theorem: This is an interesting theorem related to cyclic quadrilaterals. Let us discuss and prove it. As before, we have a cyclic quadrilateral represented by EFGH. Using Ptolemy’s theorem, which states that in cyclic quadrilateral, the product of the diagonals equals the sum of the products of pairs of two opposite sides. That is,

\((EF \times GH) + (EH \times FG) = EG \times FH\)

Or,

\({\bf{eg}} + {\bf{fh}} = {\bf{pq}}\)

This can be proven as follows. We take a cyclic quadrilateral ABCD and suppose that K is the point where its diagonals intersect. This is shown in the figure below.

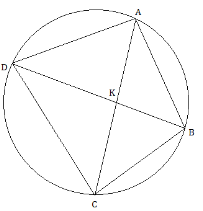
Ptolemy’s theorem

Since the angle subtended by a chord are the same at any point on the circle, we can write for chord AD, chord BC, and chord AB,

∠ABD =∠ACD

∠BCA =∠BDA

∠BAC =∠BDC

Ptolemy’s theorem

Next, we take a point E on the diagonal AC such that ∠EBC = ∠ABD. From the previous three equations, we already have ∠BCA =∠BDA and thus, we have two similar triangles, namely, triangle EBC and triangle ABD. Thus, we can write the following:

\(\begin{array}{l}\frac{{CB}}{{DB}} = \frac{{CE}}{{AD}}\\CB \times AD = CE \times DB\end{array}\)

Let us consider this equation 1 and add ∠KBE on both sides of the equation. We then get

\(\begin{array}{*{20}{c}}{\angle {\bf{EBC}}{\rm{ }} + \angle {\bf{KBE}}{\rm{ }} = \angle {\bf{ABD}}{\rm{ }} + \angle {\bf{KBE}}}\\{\angle {\bf{KBC}}{\rm{ }} = \angle {\bf{ABE}}}\end{array}\)

Ptolemy’s theorem

Similarly, we can find similar triangles BDC and ABE and do something similar, leading us to the following relations:

\(\begin{array}{l}\frac{{AB}}{{BD}} = \frac{{AE}}{{DC}}\\DC \times AB = AE \times BD\end{array}\)

We call this equation 2 and add it to equation 1 to get

\(\begin{array}{l}CB \times AD + DC \times AB = CE \times DB + AE \times BD\\CB \times AD + DC \times AB = (CE + AE) \times BD\\CB \times AD + DC \times AB = AC \times BD\end{array}\)

And thus, we have our proof.

Properties

Let’s discuss some properties regarding cyclic quadrilateral:

1. We have discussed that the opposite angles of a cyclic quadrilateral are supplementary. This is always true and thus, if the sum of opposite angles of a quadrilateral is 180⁰, then the quadrilateral is necessarily cyclic.
2. A rhombus can never be a cyclic quadrilateral since its opposite angles do not sum up to 180⁰.
3. Given a cyclic quadrilateral EFGH, with side lengths e, f, g, and h respectively, with diagonals p and q, let the diagonals intersect at a point I. We can write

\(EI \times IG = FI \times IH\)

1. Joining the midpoints of the sides of a quadrilateral gives us a parallelogram.
2. The perpendicular bisectors of the sides of a cyclic quadrilateral meet at the centre and are concurrent.

Problems and Solutions

1. In a cyclic quadrilateral EFGH, \({\bf{if}}{\rm{ }}\angle {\bf{E}}{\rm{ }} = {\rm{ }}{\bf{8}}{{\bf{5}}^{\bf{0}}},{\rm{ }}{\bf{find}}{\rm{ }}\angle {\bf{G}}\)

Since the opposite angles of a cyclic quadrilateral are supplementary, we can write

\(\begin{array}{*{20}{c}}{\angle {\bf{E}}{\rm{ }} + {\rm{ }}\angle {\bf{G}}{\rm{ }} = {\rm{ }}{\bf{18}}{{\bf{0}}^{\bf{0}}}}\\{\angle {\bf{G}}{\rm{ }} = {\rm{ }}{\bf{18}}{{\bf{0}}^{\bf{0}}}–{\rm{ }}{\bf{8}}{{\bf{5}}^{\bf{0}}} = {\rm{ }}{\bf{9}}{{\bf{5}}^{\bf{0}}}}\end{array}\)

2. Let the side lengths e, f, g, and h of a cyclic quadrilateral be 3, 6, 4, and 7m respectively. What is its area?

For a cyclic quadrilateral, the semi perimeter is given by

\(s = \frac{{e + f + g + h}}{2}\)

And the area is given by

\(A = \sqrt {(s – e) \times (s – f) \times (s – g) \times (s – h)} \)

On substituting the values, we get s = 10 m. And therefore, the area is

\(\begin{array}{l}A = \sqrt {(10 – 3)(10 – 6)(10 – 4)(10 – 7)} \\A = \sqrt {7 \times 4 \times 6 \times 3} \\A = \sqrt {504} {m^2}\end{array}\)

3. Let the side lengths of a cyclic quadrilateral be 2, 5, 3, and 6m. Find the product of the diagonals.

We can use Ptolemy’s theorem to solve this problem. We know that

\(\begin{array}{l}EF \times GH + EH \times FG = EG \times FH\\EG \times FH = 2 \times 3 + 5 \times 6 = 36\end{array}\)

Summary

This article discussed what cyclic quadrilaterals are by explaining their definition and listed a few properties related to the sides, angles, the circumcircle, the diagonals, and the area of a cyclic quadrilateral. Further, we looked at a few theorems related to such quadrilaterals, namely, Ptolemy’s theorem.

Enhance your understanding of Cyclic Quadrilaterals by enrolling in our Class 9 Maths Tuitions.

Frequently Asked Questions

1. Is every square a cyclic quadrilateral?

Yes. The sum of the opposite angles inside a square always add up to 180⁰ and therefore, all squares are cyclic in nature.

2. If we are given the lengths of sides of a cyclic quadrilateral, how do we find its diagonals?

Such problems can be solved using the properties of cyclic quadrilaterals. The diagonals p and q of a cyclic quadrilateral EFGH can be obtained via the formulae given below:

length of diagonal p \( = \sqrt {\frac{{(eg + fh) + (eh + fg)}}{{(ef + gh)}}} \)

length of diagonal q \( = \sqrt {\frac{{(eg + fh) + (ef + gh)}}{{(eh + fg)}}} \)

3. Are all parallelograms cyclic quadrilaterals?

Not necessarily. The opposite angles inside a parallelogram aren’t always supplementary and thus, may not add up to 180⁰ , which means that only some parallelograms can be cyclic.

4. How can we prove that the opposite angles of a cyclic quadrilateral are supplementary?

A: We can prove this using the fact that the opposite angles of an inscribed angle are equal. Let ABCD be a cyclic quadrilateral with center O, and let angle ABD be x and angle BCD be y. Then, angle ABC is (180 – x) degrees and angle ADC is (180 – y) degrees, since angles on a straight line add up to 180 degrees. By the inscribed angle theorem, angle ABC is equal to angle AOC, and angle ADC is equal to angle AOD. Therefore, we have:

x + y = angle ABD + angle BCD = angle AOC + angle AOD = angle AOC + (180 – angle AOC) = 180 degrees

Thus, we have shown that the opposite angles of a cyclic quadrilateral are supplementary.

5. What are some examples of real-world applications of cyclic quadrilaterals?

A: Cyclic quadrilaterals are used in a variety of fields, including engineering, architecture, and physics. For example, the design of the circular gears used in many mechanical systems is based on the properties of cyclic quadrilaterals. In architecture, the shape of many domes and arches is based on the geometry of cyclic quadrilaterals.