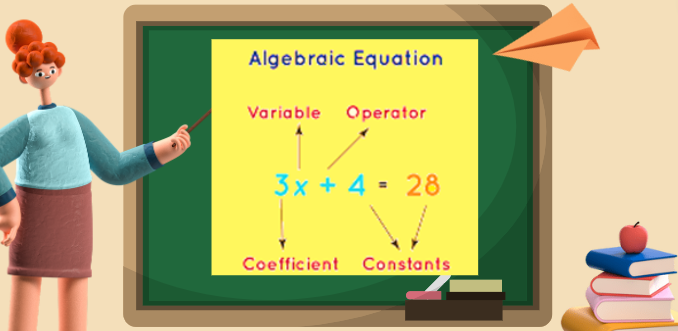
An Introduction
The value of a variable determines the value of an expression. By substituting the variable’s value in the expression and utilizing mathematical procedures, the expression is made simpler. The expression’s value is the outcome that is ultimately obtained. A whole integer, rational number, decimal number, or real number can be the value of the expression. A component of algebra is the expressions. A mathematical expression is made up of a mixture of numbers, variables, and operators. Any number of terms can combine to produce it. Any phrase may have any number of terms, ranging from 1 to n.
Find the value of the expression.
A phrase uses a variety of words. Any expression’s terms are the result of its variables and numbers. As the value of the variable changes, so do the terms’ values. Any number may be the value of the variables. As a result, the expression’s value changes together with the variable’s value. Any number of variables can be used to determine the value of the expressions. The expressions can be rationalized, factored, simplified, or enlarged to get at the answer. An expression’s value can be determined by substituting the values of its variables and then solving the expression. Only the phrasing of the expression determines its value. The value of the expression will always be a positive real number if it is the sum of two positive variables. The expression created by the product of the three negative variables will also always have a value that is a negative real number. Although it is the only possible value for the expression, the value of the variable is always a factor. The values of the variables must therefore be known in order to determine the expression’s value.
Find expression
Any expression is a concatenation of phrases related to mathematical operators +,-, etc. The terms of the expression serve as a clue. Any mathematical expression’s terms must include both numbers and variables. The English alphabets serve as a representation of the variables. Examples of the variables include, x,y,z,a,b,c and others. Defining the terms will help you find the expressions. Below are a few instances of these expressions.
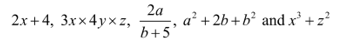
Expression in Mathematics
In algebra, expressions are one of the fundamental ideas. Expressions help you create equations, solve equations, comprehend fundamental principles of functions, and create various forms of equation solutions. Therefore, it is crucial to grasp expressions in mathematics. Expressions may contain a single variable or several variables. Mathematics uses like and unlike terms in its expressions. Finding similar and dissimilar terms in any expression is crucial since doing so simplifies complex statements. By looking at the variables in the following expressions, it is possible to determine the like terms and unlike terms of any mathematical equation. The expressions’ like terms all contain the same variables. On the other hand, there are various variables in the variable portion of the variables. For two words to be similar, their respective variable powers must be equal. Below are a few examples of mathematical expressions.
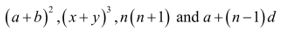
What is an expression in Mathematics?
Mathematical expressions are a collection of terms made up of variables and integers related by the addition, subtraction, multiplication, and division mathematical symbols. After learning how to calculate an expression’s value and identify an expression, explain what an expression in mathematics is. There are many identities in the study of mathematics. Two equivalent formulations make up the identity. For all possible values of the variables, the two sides of the identities are always equal. Following are explanations of a few mathematical expressions.
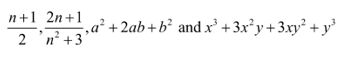
Interesting Facts
- There is never a match between the equations and the expressions.
- Expressions can be made simpler by factoring them, rationalising them, eliminating common terms from the numerator and denominator, and adding and subtracting.
- Only when the values of the variables are equal can two straightforward expressions in the same variable have values that are equal.
Solved Problems
1. Find the value for the expression a+4 when, a=2 and -2.
Sol:
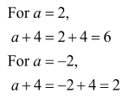
2. Calculate the value for the expression given below for x=1 and 2.

Sol:
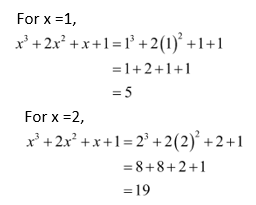
3. Simplify the expression \({y^2} + 3y\) and find its value at y = 0..
Sol: Factorize the given expression.

Summary
The expressions are used in mathematics to represent a certain value for different particular values of the respective variables. The variables can be any real values. To find the value of the expression, substitute the values of the variables and then simplify the obtained arithmetic expression. Expression is a combination of the terms with the mathematical operators. To find the expression, identify the terms and check if their combination is an expression or an equation. Expression in Mathematics is used frequently. The expression in mathematics helps to form different numbers of identities.
Practice Questions
Solve the expression x + y for x = 1, y = 2 and x = -2.5 , y = 0
Ans:
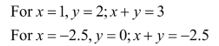
2. Simplify the expression \(\frac{{{x^3} + 2x}}{{3{x^2} + x}}\)and find its value at x =1.
Ans:
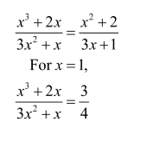
Frequently Asked Questions
1. Are the expressions and equations always the same?
Ans: No. They are not the same.
2. What is the use of expressions in mathematics?
Ans: Any general rule which is true for different sets of numbers can be represented using the expression.
3. Can two sets of variables have the same value for the expression?
Ans: Yes. Two sets of variables can have the same value for the expression.