Introduction
There are several instances when ratios and percentages are employed. The proportion and ratio ideas you acquired in earlier sessions are still fresh in your mind. On a milk packet, several milk components are listed in percentages. A certain ratio of finger millet flour and wheat flour is used to make ragi bread. To dilute a fruit juice concentrate, you must add water at a specific ratio. During the building process, a predefined ratio of cement, sand, and gravel is combined. We may calculate the percentage value of a certain amount by multiplying a ratio by \({\bf{100}}\) . In a number of computations, we also employ percentages. Let’s look at percentages and ratios in this article.
Ratios
How many times one quantity is compared to another is how a ratio is defined. The ratio should be interpreted as \({\bf{a}}\) to \({\bf{b}}\) .if it contains the values \({\bf{A}}\) and \({\bf{B}}\) in the ratio \({\bf{a}}{\rm{:}}{\bf{b}}\). This ratio may also be written as a fraction, \(\frac{{\bf{a}}}{{\bf{b}}}\) . The ratios \({\bf{1}}{\rm{:}}{\bf{2}}\), \({\bf{1}}{\rm{:}}{\bf{5}}\),and \({\bf{3}}{\rm{:}}{\bf{4}}\) are a few examples.
A ratio has two parts, antecedent and consequent. The first number is called the antecedent and the second is the consequent.
Percentage
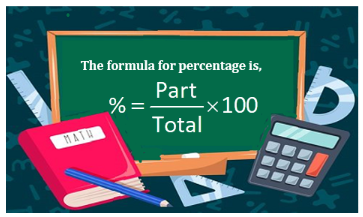
The ratio of one amount to another in terms of \({\bf{100}}\) is known as a percentage. It is a number without dimensions. The % sign is used to denote percentages.
Conversion between Ratio and Percentage
The formula below can be used to change a ratio into a percentage.
\(\% = {\bf{Ratio}} \times {\bf{100}}\)
We may use the formula below to change a percentage into a ratio.
\({\bf{Ratio}} = \frac{{{\bf{Percentage}}}}{{{\bf{100}}}}\)
Steps to Convert Data into Ratios and Percentage
Step one is to calculate the ratio of the questioned item to the total number of items.
Second step is format the ratio as a fraction.
Multiplying the fraction by \({\bf{100}}\) is step three.
Step four is to evaluate the percentage number by simplifying.
Example: Let there be \({\bf{28}}\) men and \({\bf{22}}\) women in a group of volunteers for a food camp drive.
Then the total number of volunteers is \({\bf{50}}\) .
We will find the ratio of men to women,
Ratio\( = {\rm{ }}{\bf{Men}}:{\bf{Women}}{\rm{ }} = \frac{{{\bf{Men}}}}{{{\bf{Women}}}} = \frac{{{\bf{28}}}}{{{\bf{22}}}} = \frac{{{\bf{14}}}}{{{\bf{11}}}} = {\bf{14}}:{\bf{11}}\)
Ratio of Men and Women to total is,
Ratio of Men \( = \frac{{{\bf{Men}}}}{{{\bf{Total}}}} = \frac{{{\bf{28}}}}{{{\bf{50}}}} = \frac{{{\bf{14}}}}{{{\bf{25}}}} = {\bf{14}}:{\bf{25}}\)
Ratio of Women\( = \frac{{{\bf{Women}}}}{{{\bf{Total}}}} = \frac{{{\bf{22}}}}{{{\bf{50}}}} = \frac{{{\bf{11}}}}{{{\bf{25}}}} = {\bf{11}}:{\bf{25}}\)
Finding the percentage of Men and Women respectively,
\(\% \) of Men\( = \frac{{{\bf{Men}}}}{{{\bf{Total}}}} \times {\bf{100}} = \) Ratio of Men\( \times {\bf{100}}\)
\( = \frac{{{\bf{14}}}}{{{\bf{25}}}} \times {\bf{100}} = {\bf{56}}\% \)
\(\% \) of Women\( = \frac{{{\bf{Men}}}}{{{\bf{Total}}}} \times {\bf{100}} = \) Ratio of Women\( \times {\bf{100}}\)
\( = \frac{{{\bf{11}}}}{{{\bf{25}}}} \times {\bf{100}} = {\bf{44}}\% \)
Applications of Ratio and Percentage
- When combining two ingredients, ratios can be used. For instance, combining two different types of wheat and creating solutions with various liquids.
- Government authorities employ percentages when making some crucial choices, such those regarding assistance programms.
- More data may be simply understood and interpreted by us.
- Percentages are used by researchers to compare data.
- In order to calculate profit and loss and to understand how the shares of various stakeholders in a firm contribute, businesspeople utilize percentages.
- When developing a township or building a home, engineers allocate regions using percentages.
Difference between Ratio and Percentage
| Ratio | Percentage |
| How many times one quantity is compared to another is how a ratio is defined. The ratio should be interpreted as a to b if it contains the values A and B in the ratio a:b. | The ratio of one amount to another in terms of 100 is known as a percentage. It is a number without dimensions. The % sign is used to denote percentages. |
| Ratios are comparisons between two parts of the same thing. | The percentage represents the part of the whole in terms of 100 parts, i.e., the number of sections the part has if the whole is divided into 100 sections. |
| Example: 1:2, 3:5 and 7:4, etc. | Example: 50%, 20% and 125% etc. |
Interesting Facts
- The ratio of the Earth’s diameter to the Sun’s is \({\bf{1}}:{\bf{108}}\) .
- To change one currency into another, one uses ratios.
- The ratio between the heights and bases of similar triangles is \({\bf{1}}\) .
Solved Examples
Example: Find the ratio of Ravi and Suraj’s income, if Ravi earns \({\bf{40}}\% \) more than Suraj.
Ans: Let Suraj’s income be \({\bf{x}}\) .
Then Ravi’s income \( = {\bf{x}} + {\bf{40}}\% {\rm{ }}{\bf{of}}{\rm{ }}{\bf{x}}\)
\( = {\bf{x}} + \frac{{{\bf{40}}}}{{{\bf{100}}}}{\bf{x}} = \frac{{{\bf{140x}}}}{{{\bf{100}}}} = \frac{{{\bf{7x}}}}{{\bf{5}}}\)
Then the ratio of Ravi’s and Suraj’s income = Ravi’s Income: Suraj’s Income
\( = \frac{{{\bf{7x}}}}{{\bf{5}}}:{\bf{x}}\)
\( = {\bf{7}}:{\bf{5}}\)
Summary
In this article, we have learned about ratios and percentages. A ratio is the proportion of one quantity to another. The ratio of one quantity to another, stated in terms of \({\bf{100}}\) , is known as a percentage. For comparing amounts, we employ percentages as well as ratios.
Frequently Asked Questions
1. What are equivalent ratios?
Ans. Equivalent ratios are defined as the ratios comparing two different pair of quantities which have the same overall value, these ratios do not look the same on the first view, but on some mathematical manipulations they become exactly the same.
\({\bf{1}}:{\bf{2}},{\bf{2}}:{\bf{4}},{\bf{3}}:{\bf{6}}\) are all equivalent ratios to each other.
2. Which is more important, ratios or percentages?
Ans. To compare quantities with the whole, we use percentages and to compare two parts of the same thing we use ratios. Both have their own different use, and both are equally important for that. But we cannot compare quantities using percentages if the whole quantity is not given to us, but we can directly compare ratios to compare two parts without the knowledge of the whole. Which is why the ratios are slightly more important than percentages while comparing quantities. Whereas in practicality percentage are more easier to understand than ratios, which is why percentages are seen more often in real life examples, such as a discount of \({\bf{25}}\% \) is easier to interpret rather than saying a discount of \(\;{\bf{1}}:{\bf{4}}\).
3. What are proportions?
Ans. Proportions are a comparison between two ratios that are comparing two different pairs of quantities. They are represented by the symbol, ‘\(::\)’.